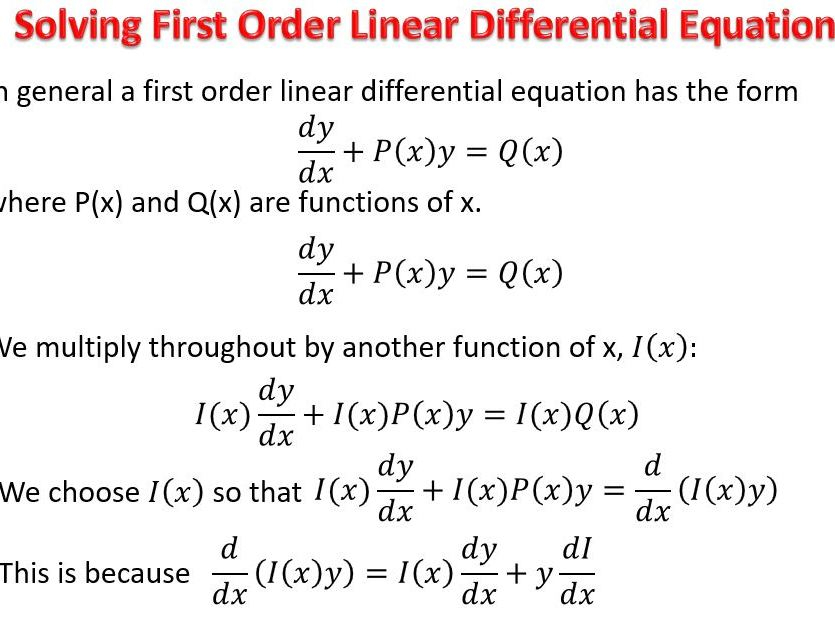
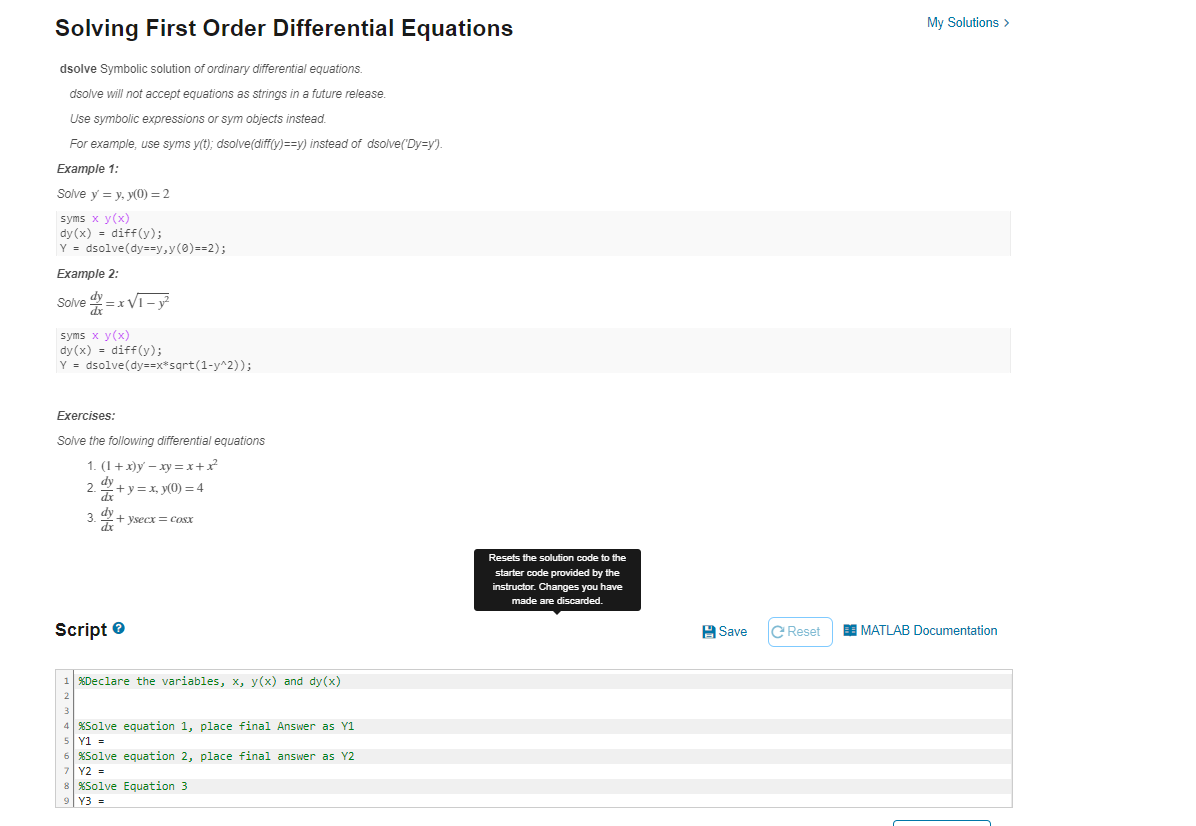
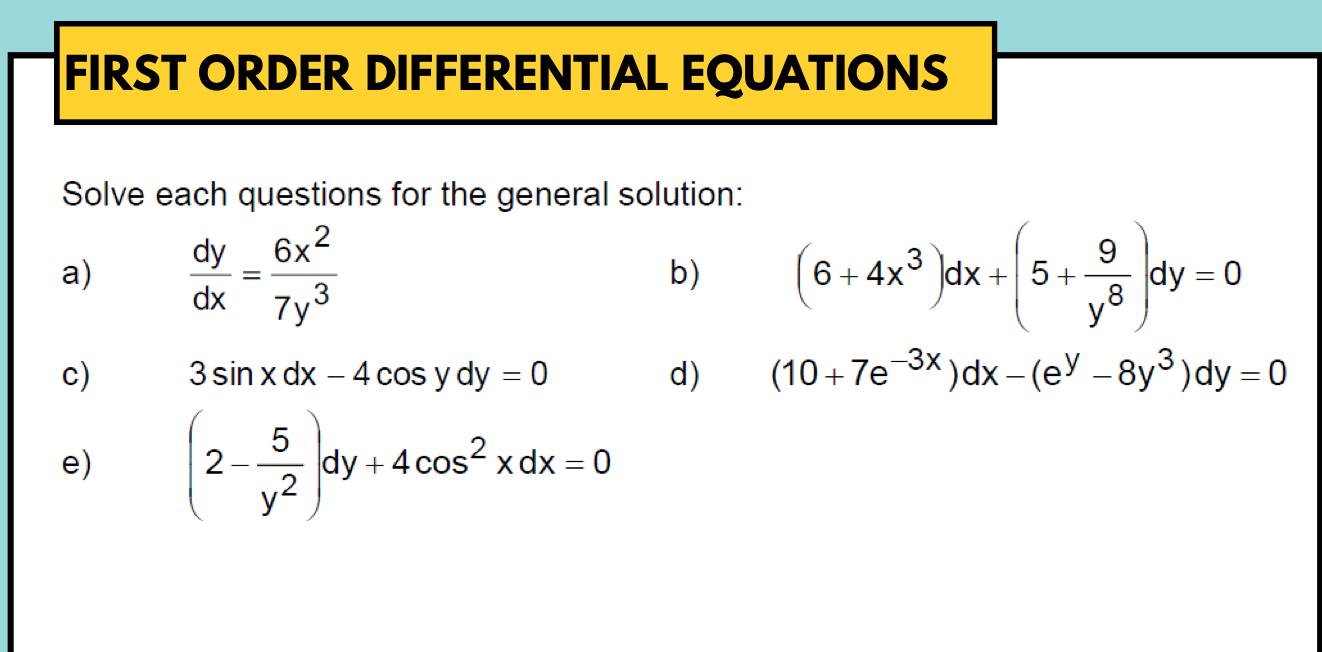
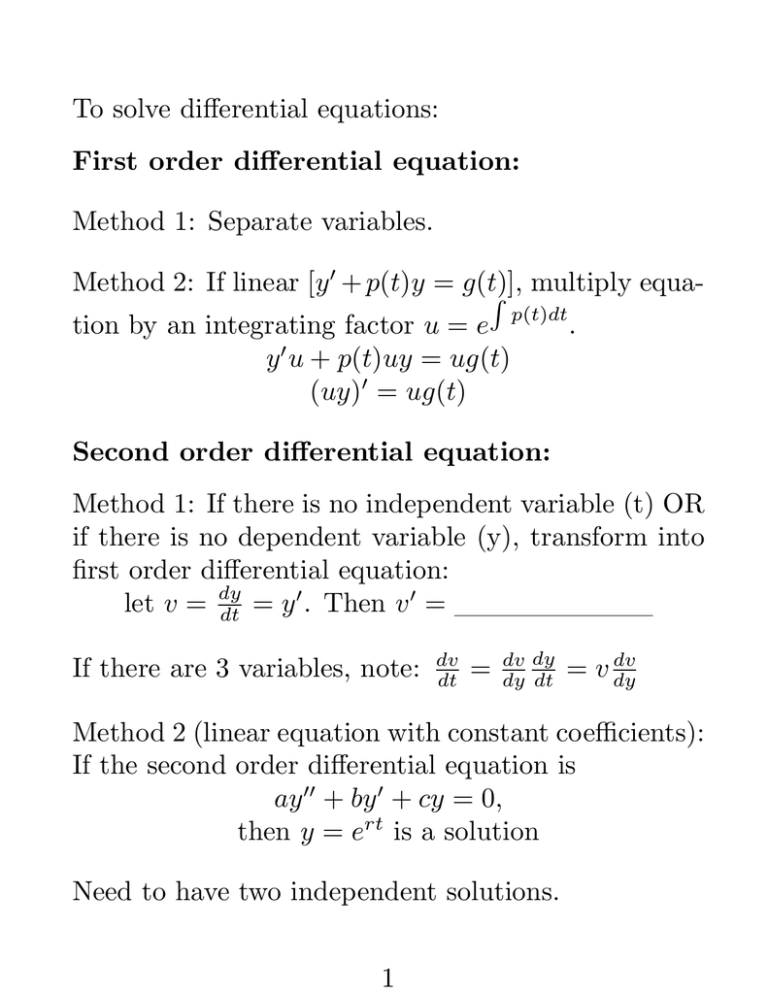
How To Solve First Order Differential Equations - A first order differential equation is an equation of the form \(f(t, y, \dot{y})=0\). A solution of a first order differential equation is a function \(f(t)\) that makes \(f(t,f(t),f'(t))=0\) for. In a level maths you will have solved some first order differential equations using the method of separation of variables A first order differential equation is linear when it can be made to look like this: In this chapter we will look at several of the standard solution methods for first order differential equations including linear, separable, exact and bernoulli differential equations. Dy dx + p(x)y = q(x) where p(x) and q(x) are functions of x. To solve it there is a special method:
A first order differential equation is an equation of the form \(f(t, y, \dot{y})=0\). A first order differential equation is linear when it can be made to look like this: In this chapter we will look at several of the standard solution methods for first order differential equations including linear, separable, exact and bernoulli differential equations. In a level maths you will have solved some first order differential equations using the method of separation of variables To solve it there is a special method: Dy dx + p(x)y = q(x) where p(x) and q(x) are functions of x. A solution of a first order differential equation is a function \(f(t)\) that makes \(f(t,f(t),f'(t))=0\) for.
A first order differential equation is linear when it can be made to look like this: A solution of a first order differential equation is a function \(f(t)\) that makes \(f(t,f(t),f'(t))=0\) for. In a level maths you will have solved some first order differential equations using the method of separation of variables Dy dx + p(x)y = q(x) where p(x) and q(x) are functions of x. To solve it there is a special method: In this chapter we will look at several of the standard solution methods for first order differential equations including linear, separable, exact and bernoulli differential equations. A first order differential equation is an equation of the form \(f(t, y, \dot{y})=0\).
Linear FirstOrder Differential Equations
In a level maths you will have solved some first order differential equations using the method of separation of variables A first order differential equation is linear when it can be made to look like this: To solve it there is a special method: A solution of a first order differential equation is a function \(f(t)\) that makes \(f(t,f(t),f'(t))=0\) for..
First Order Differential Equation Worksheet Equations Worksheets
To solve it there is a special method: A first order differential equation is an equation of the form \(f(t, y, \dot{y})=0\). A first order differential equation is linear when it can be made to look like this: A solution of a first order differential equation is a function \(f(t)\) that makes \(f(t,f(t),f'(t))=0\) for. Dy dx + p(x)y = q(x).
Solved Solving First Order Differential Equations dsolve
To solve it there is a special method: A solution of a first order differential equation is a function \(f(t)\) that makes \(f(t,f(t),f'(t))=0\) for. A first order differential equation is an equation of the form \(f(t, y, \dot{y})=0\). In this chapter we will look at several of the standard solution methods for first order differential equations including linear, separable, exact.
Solved Solving First Order Differential Equations My
A first order differential equation is an equation of the form \(f(t, y, \dot{y})=0\). A solution of a first order differential equation is a function \(f(t)\) that makes \(f(t,f(t),f'(t))=0\) for. In this chapter we will look at several of the standard solution methods for first order differential equations including linear, separable, exact and bernoulli differential equations. In a level maths.
Solved FIRST ORDER DIFFERENTIAL EQUATIONSSolve each
In this chapter we will look at several of the standard solution methods for first order differential equations including linear, separable, exact and bernoulli differential equations. In a level maths you will have solved some first order differential equations using the method of separation of variables A solution of a first order differential equation is a function \(f(t)\) that makes.
SOLUTION First order differential equations Studypool
To solve it there is a special method: A first order differential equation is an equation of the form \(f(t, y, \dot{y})=0\). A first order differential equation is linear when it can be made to look like this: Dy dx + p(x)y = q(x) where p(x) and q(x) are functions of x. In a level maths you will have solved.
To solve differential equations First order differential equation
Dy dx + p(x)y = q(x) where p(x) and q(x) are functions of x. In a level maths you will have solved some first order differential equations using the method of separation of variables A solution of a first order differential equation is a function \(f(t)\) that makes \(f(t,f(t),f'(t))=0\) for. A first order differential equation is linear when it can.
SOLUTION First order differential equations Studypool
To solve it there is a special method: A first order differential equation is an equation of the form \(f(t, y, \dot{y})=0\). Dy dx + p(x)y = q(x) where p(x) and q(x) are functions of x. In this chapter we will look at several of the standard solution methods for first order differential equations including linear, separable, exact and bernoulli.
How to Solve separable firstorder differential equations « Math
To solve it there is a special method: A first order differential equation is an equation of the form \(f(t, y, \dot{y})=0\). In this chapter we will look at several of the standard solution methods for first order differential equations including linear, separable, exact and bernoulli differential equations. In a level maths you will have solved some first order differential.
1.2. First Order Differential Equations PDF Equations
In a level maths you will have solved some first order differential equations using the method of separation of variables A first order differential equation is linear when it can be made to look like this: To solve it there is a special method: In this chapter we will look at several of the standard solution methods for first order.
Dy Dx + P(X)Y = Q(X) Where P(X) And Q(X) Are Functions Of X.
A first order differential equation is linear when it can be made to look like this: To solve it there is a special method: A solution of a first order differential equation is a function \(f(t)\) that makes \(f(t,f(t),f'(t))=0\) for. A first order differential equation is an equation of the form \(f(t, y, \dot{y})=0\).
In A Level Maths You Will Have Solved Some First Order Differential Equations Using The Method Of Separation Of Variables
In this chapter we will look at several of the standard solution methods for first order differential equations including linear, separable, exact and bernoulli differential equations.