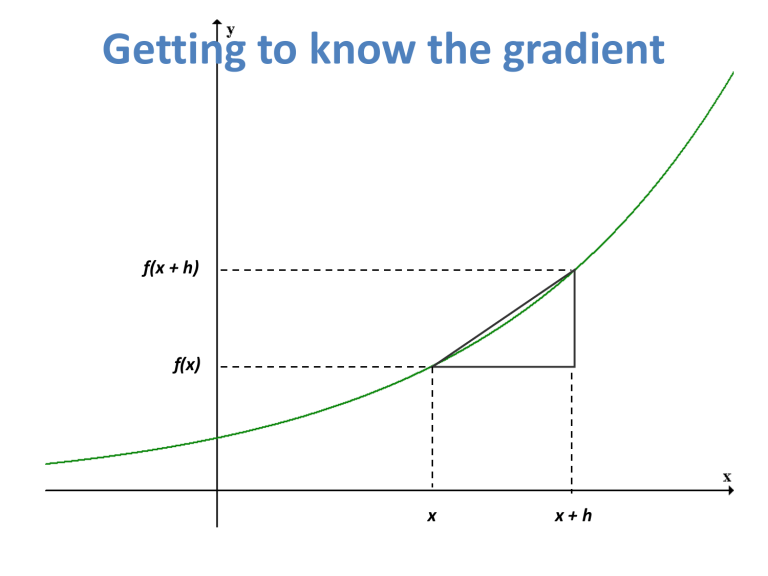
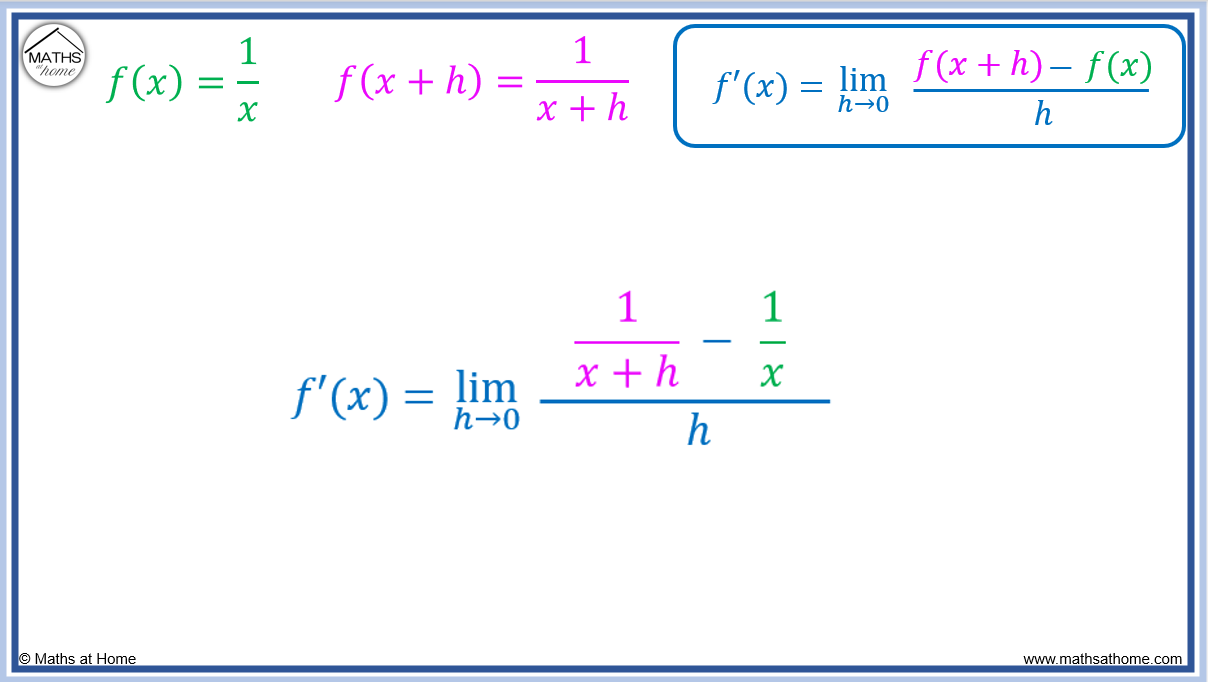
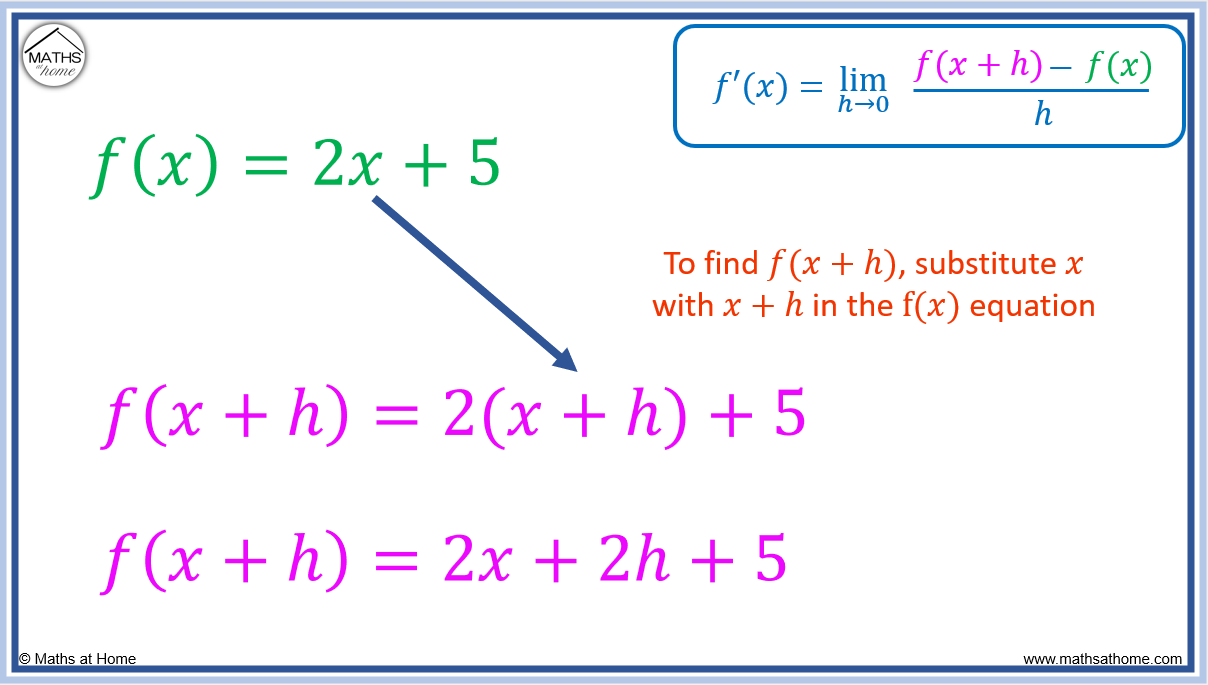
Differentiation From 1St Principles - Use differentiation from first principles to find the gradient function of y = 1. Find the derivative of the following, using differentiation from first. Give increments to both x & y i.e. Find rate of change of. 4 steps to work out differentiation from the first principle:
4 steps to work out differentiation from the first principle: Give increments to both x & y i.e. Find the derivative of the following, using differentiation from first. Find rate of change of. Use differentiation from first principles to find the gradient function of y = 1.
Find the derivative of the following, using differentiation from first. Give increments to both x & y i.e. Find rate of change of. 4 steps to work out differentiation from the first principle: Use differentiation from first principles to find the gradient function of y = 1.
How to Differentiate by First Principles
4 steps to work out differentiation from the first principle: Use differentiation from first principles to find the gradient function of y = 1. Give increments to both x & y i.e. Find rate of change of. Find the derivative of the following, using differentiation from first.
How to Differentiate by First Principles
Use differentiation from first principles to find the gradient function of y = 1. Find rate of change of. Find the derivative of the following, using differentiation from first. Give increments to both x & y i.e. 4 steps to work out differentiation from the first principle:
Differentiation from 1st principles Teaching Resources
4 steps to work out differentiation from the first principle: Find the derivative of the following, using differentiation from first. Give increments to both x & y i.e. Find rate of change of. Use differentiation from first principles to find the gradient function of y = 1.
Differentiation from first principles
Use differentiation from first principles to find the gradient function of y = 1. Find the derivative of the following, using differentiation from first. Give increments to both x & y i.e. Find rate of change of. 4 steps to work out differentiation from the first principle:
1st Principles TYS 1 solns
Find the derivative of the following, using differentiation from first. Give increments to both x & y i.e. Find rate of change of. 4 steps to work out differentiation from the first principle: Use differentiation from first principles to find the gradient function of y = 1.
Differentiation from 1st principles
Find the derivative of the following, using differentiation from first. Find rate of change of. 4 steps to work out differentiation from the first principle: Give increments to both x & y i.e. Use differentiation from first principles to find the gradient function of y = 1.
How to Differentiate by First Principles
4 steps to work out differentiation from the first principle: Give increments to both x & y i.e. Find rate of change of. Use differentiation from first principles to find the gradient function of y = 1. Find the derivative of the following, using differentiation from first.
How to Differentiate by First Principles
Use differentiation from first principles to find the gradient function of y = 1. Find the derivative of the following, using differentiation from first. 4 steps to work out differentiation from the first principle: Give increments to both x & y i.e. Find rate of change of.
How to Differentiate by First Principles
Find rate of change of. Use differentiation from first principles to find the gradient function of y = 1. 4 steps to work out differentiation from the first principle: Find the derivative of the following, using differentiation from first. Give increments to both x & y i.e.
How to Differentiate by First Principles
Use differentiation from first principles to find the gradient function of y = 1. 4 steps to work out differentiation from the first principle: Find rate of change of. Find the derivative of the following, using differentiation from first. Give increments to both x & y i.e.
Use Differentiation From First Principles To Find The Gradient Function Of Y = 1.
4 steps to work out differentiation from the first principle: Give increments to both x & y i.e. Find the derivative of the following, using differentiation from first. Find rate of change of.