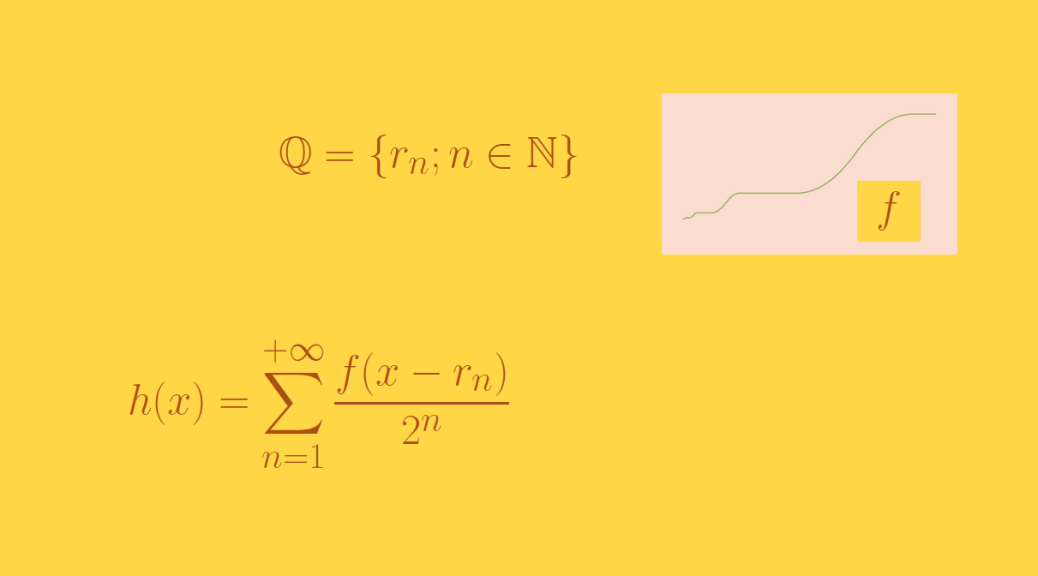Totally Differentiable - The former part of δ x is called the (total) differential or the exact differential of the function f in the point (x, y, z) and it is denoted. Let \(w=f(x,y,z)\) be continuous on an open set \(s\). The total differential gives an approximation of the change in z given small changes in x and y. We can use this to approximate error propagation;. Let \(dx\), \(dy\) and \(dz\) represent changes. Total differentials can be generalized. For a function f = f(x,y,z) whose partial derivatives exists, the total differential of f is given by df = f.
Total differentials can be generalized. Let \(w=f(x,y,z)\) be continuous on an open set \(s\). The total differential gives an approximation of the change in z given small changes in x and y. We can use this to approximate error propagation;. The former part of δ x is called the (total) differential or the exact differential of the function f in the point (x, y, z) and it is denoted. For a function f = f(x,y,z) whose partial derivatives exists, the total differential of f is given by df = f. Let \(dx\), \(dy\) and \(dz\) represent changes.
Let \(w=f(x,y,z)\) be continuous on an open set \(s\). We can use this to approximate error propagation;. The total differential gives an approximation of the change in z given small changes in x and y. For a function f = f(x,y,z) whose partial derivatives exists, the total differential of f is given by df = f. Total differentials can be generalized. The former part of δ x is called the (total) differential or the exact differential of the function f in the point (x, y, z) and it is denoted. Let \(dx\), \(dy\) and \(dz\) represent changes.
A continuous function not differentiable at the rationals but
The former part of δ x is called the (total) differential or the exact differential of the function f in the point (x, y, z) and it is denoted. Total differentials can be generalized. Let \(w=f(x,y,z)\) be continuous on an open set \(s\). Let \(dx\), \(dy\) and \(dz\) represent changes. The total differential gives an approximation of the change.
Continuous but Nowhere Differentiable Math Fun Facts
The total differential gives an approximation of the change in z given small changes in x and y. Let \(dx\), \(dy\) and \(dz\) represent changes. The former part of δ x is called the (total) differential or the exact differential of the function f in the point (x, y, z) and it is denoted. We can use this to.
Differentiable vs. Continuous Functions Understanding the Distinctions
Let \(dx\), \(dy\) and \(dz\) represent changes. For a function f = f(x,y,z) whose partial derivatives exists, the total differential of f is given by df = f. Let \(w=f(x,y,z)\) be continuous on an open set \(s\). Total differentials can be generalized. The total differential gives an approximation of the change in z given small changes in x and y.
Solved A5. Prove that if f D → R2 is totally
Let \(dx\), \(dy\) and \(dz\) represent changes. The former part of δ x is called the (total) differential or the exact differential of the function f in the point (x, y, z) and it is denoted. Let \(w=f(x,y,z)\) be continuous on an open set \(s\). For a function f = f(x,y,z) whose partial derivatives exists, the total differential of.
Differentiable Font download free
We can use this to approximate error propagation;. The total differential gives an approximation of the change in z given small changes in x and y. For a function f = f(x,y,z) whose partial derivatives exists, the total differential of f is given by df = f. Let \(w=f(x,y,z)\) be continuous on an open set \(s\). Let \(dx\), \(dy\) and.
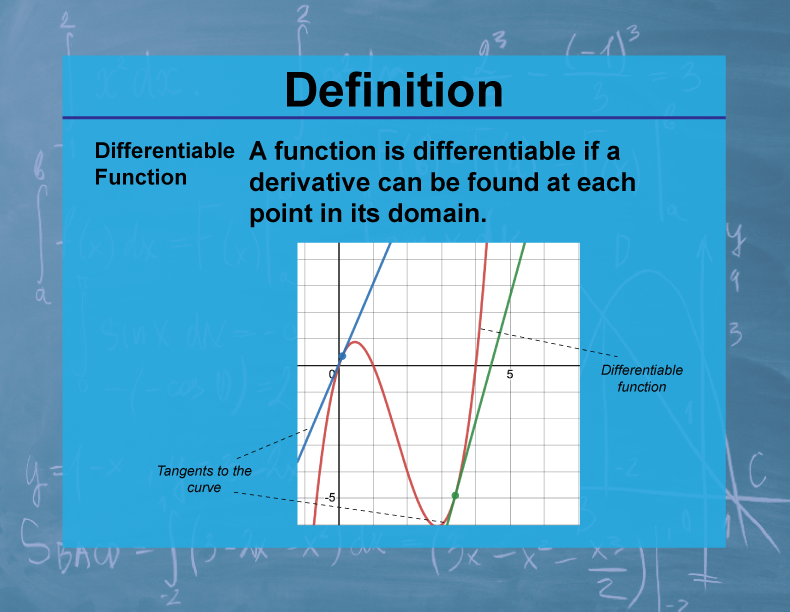
DefinitionCalculus TopicsDifferentiable Function Media4Math
Let \(w=f(x,y,z)\) be continuous on an open set \(s\). The former part of δ x is called the (total) differential or the exact differential of the function f in the point (x, y, z) and it is denoted. We can use this to approximate error propagation;. For a function f = f(x,y,z) whose partial derivatives exists, the total differential.
Differentiable function Wikiwand
Total differentials can be generalized. The former part of δ x is called the (total) differential or the exact differential of the function f in the point (x, y, z) and it is denoted. Let \(dx\), \(dy\) and \(dz\) represent changes. For a function f = f(x,y,z) whose partial derivatives exists, the total differential of f is given by.
PPT Differentiable functions are Continuous PowerPoint Presentation
The total differential gives an approximation of the change in z given small changes in x and y. Total differentials can be generalized. The former part of δ x is called the (total) differential or the exact differential of the function f in the point (x, y, z) and it is denoted. Let \(dx\), \(dy\) and \(dz\) represent changes..
Differentiable Programming A Simple Introduction
Total differentials can be generalized. For a function f = f(x,y,z) whose partial derivatives exists, the total differential of f is given by df = f. The former part of δ x is called the (total) differential or the exact differential of the function f in the point (x, y, z) and it is denoted. Let \(w=f(x,y,z)\) be continuous.
Differentiable Turbulence Paper and Code CatalyzeX
Let \(w=f(x,y,z)\) be continuous on an open set \(s\). The total differential gives an approximation of the change in z given small changes in x and y. For a function f = f(x,y,z) whose partial derivatives exists, the total differential of f is given by df = f. Total differentials can be generalized. The former part of δ x.
The Total Differential Gives An Approximation Of The Change In Z Given Small Changes In X And Y.
For a function f = f(x,y,z) whose partial derivatives exists, the total differential of f is given by df = f. Total differentials can be generalized. The former part of δ x is called the (total) differential or the exact differential of the function f in the point (x, y, z) and it is denoted. We can use this to approximate error propagation;.
Let \(W=F(X,Y,Z)\) Be Continuous On An Open Set \(S\).
Let \(dx\), \(dy\) and \(dz\) represent changes.