Total Differentiation

Total Differentiation - See examples, exercises and solutions for functions of two. Use the mean value theorem to express each difference in terms of a partial derivative of f times a coordinate of x − p (both. Let $w=f(x,y,z)$ be continuous on an open set $s$. Learn how to calculate total differentials and use them to approximate functions. Let $dx$, $dy$ and $dz$ represent changes. Learn how to compute and evaluate the total differential of a function of several variables using the chain rule and the tangent approximation.

Let $w=f(x,y,z)$ be continuous on an open set $s$. See examples, exercises and solutions for functions of two. Let $dx$, $dy$ and $dz$ represent changes. Learn how to calculate total differentials and use them to approximate functions. Learn how to compute and evaluate the total differential of a function of several variables using the chain rule and the tangent approximation. Use the mean value theorem to express each difference in terms of a partial derivative of f times a coordinate of x − p (both.

Learn how to compute and evaluate the total differential of a function of several variables using the chain rule and the tangent approximation. Learn how to calculate total differentials and use them to approximate functions. Let $w=f(x,y,z)$ be continuous on an open set $s$. See examples, exercises and solutions for functions of two. Use the mean value theorem to express each difference in terms of a partial derivative of f times a coordinate of x − p (both. Let $dx$, $dy$ and $dz$ represent changes.

Partial Differentiation Definition & Rules Lesson

Learn how to compute and evaluate the total differential of a function of several variables using the chain rule and the tangent approximation. Let $dx$, $dy$ and $dz$ represent changes. Let $w=f(x,y,z)$ be continuous on an open set $s$. Use the mean value theorem to express each difference in terms of a partial derivative of f times a coordinate of.

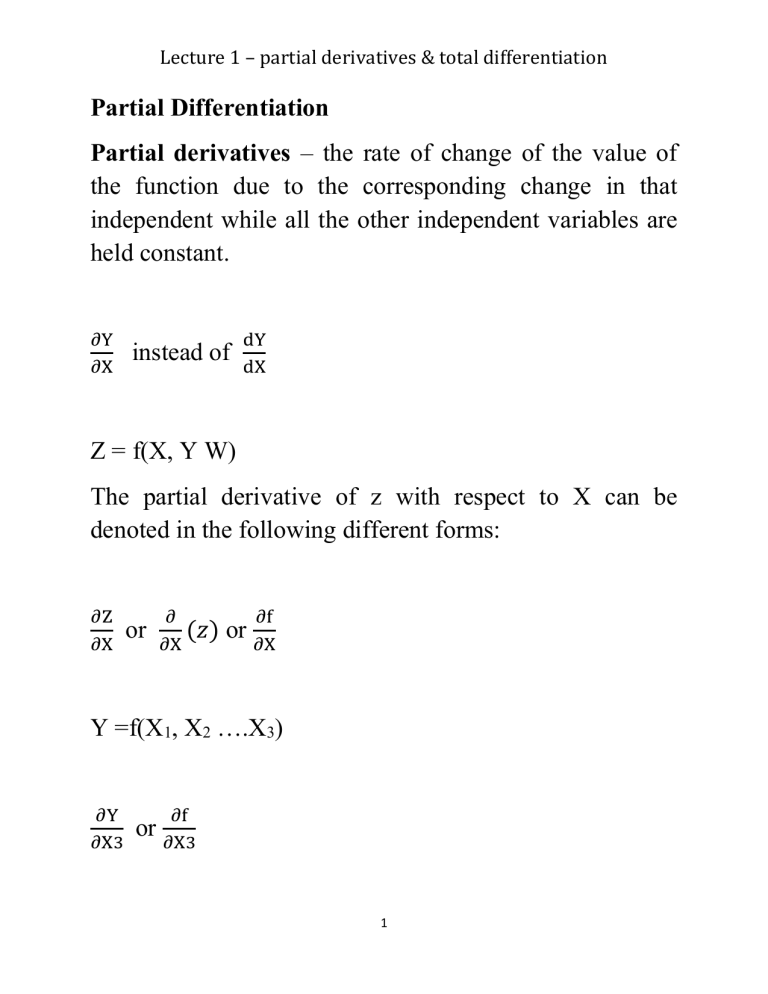
Total Differentiation of a Vector in a Rotating Frame of Reference

Let $dx$, $dy$ and $dz$ represent changes. Learn how to compute and evaluate the total differential of a function of several variables using the chain rule and the tangent approximation. Use the mean value theorem to express each difference in terms of a partial derivative of f times a coordinate of x − p (both. Let $w=f(x,y,z)$ be continuous on.

Total differentiation,partial differentiation. Docsity

Use the mean value theorem to express each difference in terms of a partial derivative of f times a coordinate of x − p (both. Learn how to compute and evaluate the total differential of a function of several variables using the chain rule and the tangent approximation. See examples, exercises and solutions for functions of two. Let $w=f(x,y,z)$ be.

$What Is The Differentiation Process For [math]\ln(x), 51 OFF$

What Is The Differentiation Process For [math]\ln(x), 51 OFF

Let $dx$, $dy$ and $dz$ represent changes. Let $w=f(x,y,z)$ be continuous on an open set $s$. Learn how to compute and evaluate the total differential of a function of several variables using the chain rule and the tangent approximation. Use the mean value theorem to express each difference in terms of a partial derivative of f times a coordinate of.

(PDF) Chap. 12 Differentiation and total differentiation · total

Let $dx$, $dy$ and $dz$ represent changes. Use the mean value theorem to express each difference in terms of a partial derivative of f times a coordinate of x − p (both. Learn how to compute and evaluate the total differential of a function of several variables using the chain rule and the tangent approximation. Let $w=f(x,y,z)$ be continuous on.

calculus Problem with Total differentiation Mathematics Stack Exchange

See examples, exercises and solutions for functions of two. Let $w=f(x,y,z)$ be continuous on an open set $s$. Let $dx$, $dy$ and $dz$ represent changes. Use the mean value theorem to express each difference in terms of a partial derivative of f times a coordinate of x − p (both. Learn how to compute and evaluate the total differential of.

differentiation Irish Primary Teacher

Let $dx$, $dy$ and $dz$ represent changes. See examples, exercises and solutions for functions of two. Learn how to compute and evaluate the total differential of a function of several variables using the chain rule and the tangent approximation. Learn how to calculate total differentials and use them to approximate functions. Use the mean value theorem to express each difference.

Total Differentiation and Composite Functions Examples PDF

Learn how to calculate total differentials and use them to approximate functions. Let $w=f(x,y,z)$ be continuous on an open set $s$. Let $dx$, $dy$ and $dz$ represent changes. Learn how to compute and evaluate the total differential of a function of several variables using the chain rule and the tangent approximation. Use the mean value theorem to express each difference.

calculus Partial differentiation total differential Mathematics

Use the mean value theorem to express each difference in terms of a partial derivative of f times a coordinate of x − p (both. Let $w=f(x,y,z)$ be continuous on an open set $s$. Let $dx$, $dy$ and $dz$ represent changes. Learn how to compute and evaluate the total differential of a function of several variables using the chain rule.

Lecture 1 partial Derivatives & Total Differentiation

Learn how to calculate total differentials and use them to approximate functions. Let $w=f(x,y,z)$ be continuous on an open set $s$. Let $dx$, $dy$ and $dz$ represent changes. Use the mean value theorem to express each difference in terms of a partial derivative of f times a coordinate of x − p (both. See examples, exercises and solutions for functions.

See Examples, Exercises And Solutions For Functions Of Two.

Use the mean value theorem to express each difference in terms of a partial derivative of f times a coordinate of x − p (both. Let $dx$, $dy$ and $dz$ represent changes. Learn how to compute and evaluate the total differential of a function of several variables using the chain rule and the tangent approximation. Learn how to calculate total differentials and use them to approximate functions.