System Of Linear Differential Equations - A system of linear differential equations is a set of linear equations relating a group of functions to their derivatives. If a(t) is an n n matrix function that is. A linear system takes the form x0 = a(t)x +b(t)y +e(t) y0 = c(t)x +d(t)y + f(t).(6.7) a homogeneous linear system results when e(t) = 0 and f(t) = 0. In this section we will look at some of the basics of systems of differential equations. As with linear systems, a homogeneous linear system of di erential equations is one in which b(t) = 0. Section 10.3 deals with the basic theory of homogeneous. We show how to convert a system of. Section 10.2 discusses linear systems of differential equations.
We show how to convert a system of. If a(t) is an n n matrix function that is. Section 10.3 deals with the basic theory of homogeneous. In this section we will look at some of the basics of systems of differential equations. A system of linear differential equations is a set of linear equations relating a group of functions to their derivatives. As with linear systems, a homogeneous linear system of di erential equations is one in which b(t) = 0. Section 10.2 discusses linear systems of differential equations. A linear system takes the form x0 = a(t)x +b(t)y +e(t) y0 = c(t)x +d(t)y + f(t).(6.7) a homogeneous linear system results when e(t) = 0 and f(t) = 0.
As with linear systems, a homogeneous linear system of di erential equations is one in which b(t) = 0. A linear system takes the form x0 = a(t)x +b(t)y +e(t) y0 = c(t)x +d(t)y + f(t).(6.7) a homogeneous linear system results when e(t) = 0 and f(t) = 0. In this section we will look at some of the basics of systems of differential equations. We show how to convert a system of. Section 10.2 discusses linear systems of differential equations. Section 10.3 deals with the basic theory of homogeneous. If a(t) is an n n matrix function that is. A system of linear differential equations is a set of linear equations relating a group of functions to their derivatives.
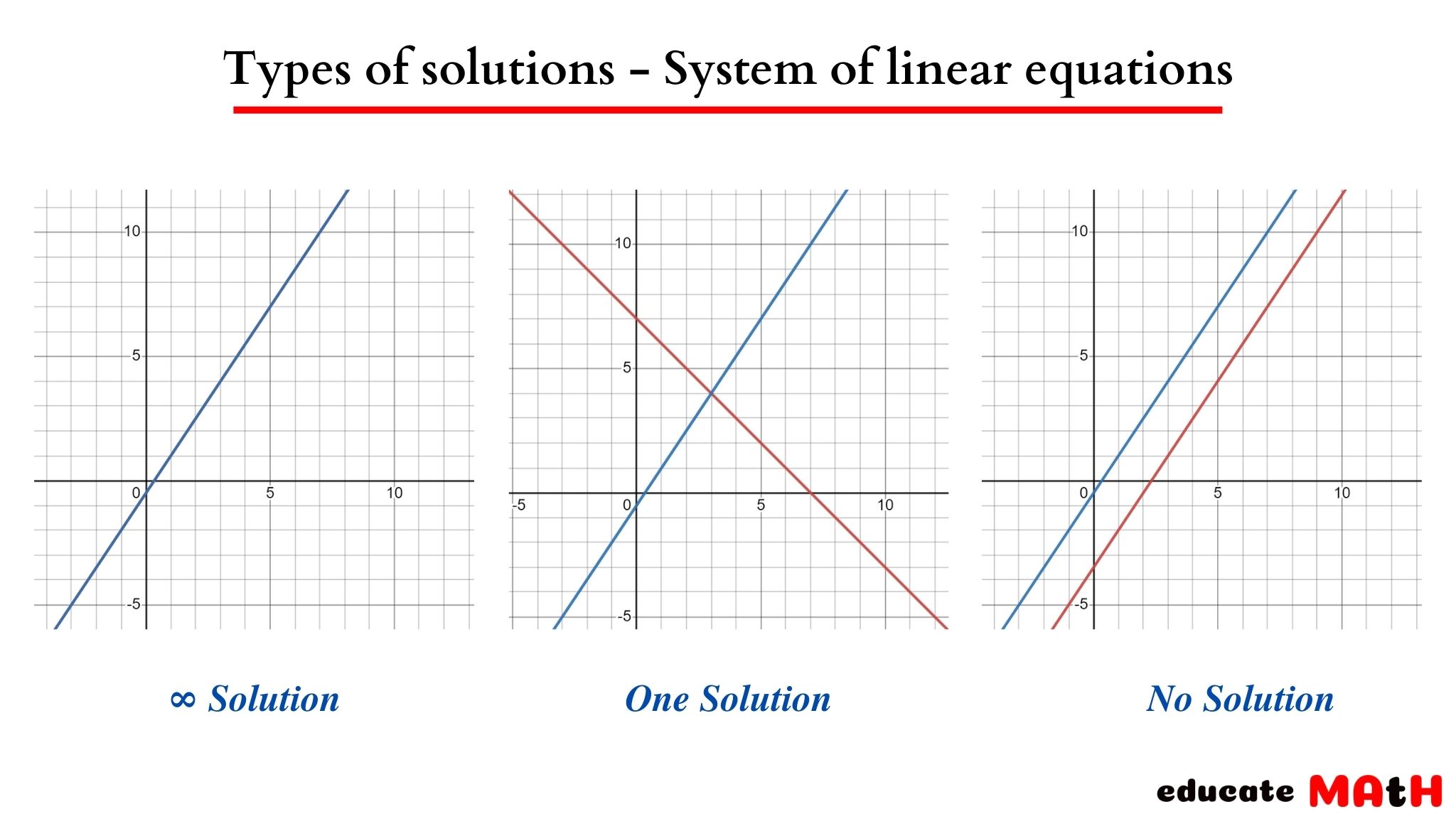
How to Solve a System of Linear Equations
As with linear systems, a homogeneous linear system of di erential equations is one in which b(t) = 0. If a(t) is an n n matrix function that is. Section 10.2 discusses linear systems of differential equations. A linear system takes the form x0 = a(t)x +b(t)y +e(t) y0 = c(t)x +d(t)y + f(t).(6.7) a homogeneous linear system results when.
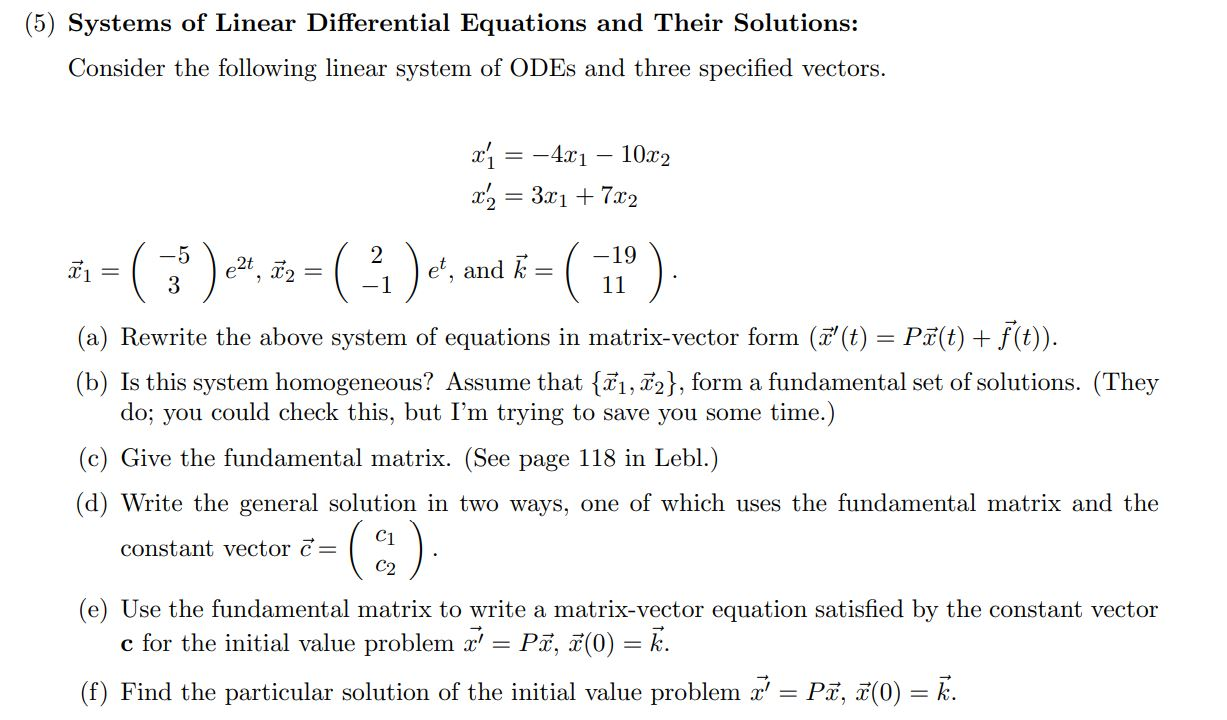
Solved (5) Systems of Linear Differential Equations and
As with linear systems, a homogeneous linear system of di erential equations is one in which b(t) = 0. We show how to convert a system of. A linear system takes the form x0 = a(t)x +b(t)y +e(t) y0 = c(t)x +d(t)y + f(t).(6.7) a homogeneous linear system results when e(t) = 0 and f(t) = 0. A system of.
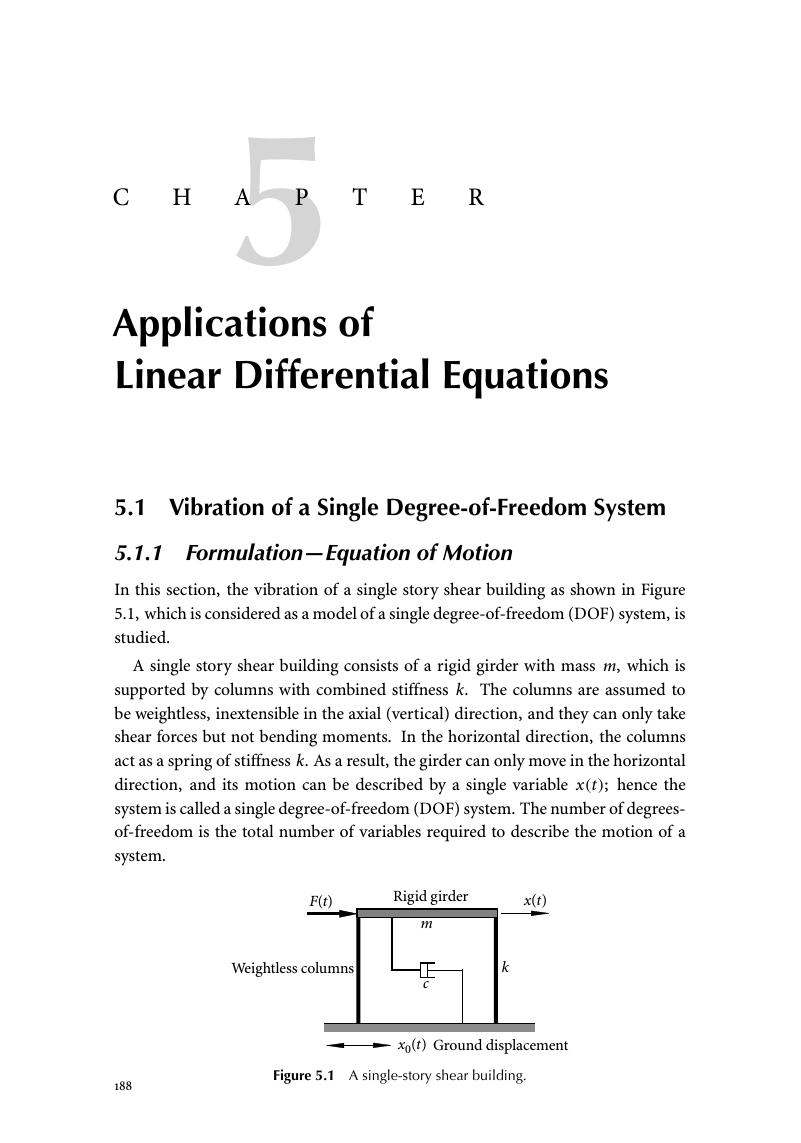
Applications of Linear Differential Equations (Chapter 5
We show how to convert a system of. Section 10.2 discusses linear systems of differential equations. In this section we will look at some of the basics of systems of differential equations. A linear system takes the form x0 = a(t)x +b(t)y +e(t) y0 = c(t)x +d(t)y + f(t).(6.7) a homogeneous linear system results when e(t) = 0 and f(t).
Chapter 3 Linear Differential Equation PDF Equations Differential
In this section we will look at some of the basics of systems of differential equations. Section 10.3 deals with the basic theory of homogeneous. As with linear systems, a homogeneous linear system of di erential equations is one in which b(t) = 0. A linear system takes the form x0 = a(t)x +b(t)y +e(t) y0 = c(t)x +d(t)y +.
SOLUTION Simultaneous linear differential equations Studypool
Section 10.3 deals with the basic theory of homogeneous. Section 10.2 discusses linear systems of differential equations. We show how to convert a system of. In this section we will look at some of the basics of systems of differential equations. A system of linear differential equations is a set of linear equations relating a group of functions to their.
linear differential equations and applications Shop Handwritten Notes
If a(t) is an n n matrix function that is. In this section we will look at some of the basics of systems of differential equations. As with linear systems, a homogeneous linear system of di erential equations is one in which b(t) = 0. A linear system takes the form x0 = a(t)x +b(t)y +e(t) y0 = c(t)x +d(t)y.
SOLUTION First order linear differential equations Studypool
As with linear systems, a homogeneous linear system of di erential equations is one in which b(t) = 0. If a(t) is an n n matrix function that is. In this section we will look at some of the basics of systems of differential equations. A system of linear differential equations is a set of linear equations relating a group.
SOLUTION Notes on linear differential equations Studypool
As with linear systems, a homogeneous linear system of di erential equations is one in which b(t) = 0. We show how to convert a system of. A system of linear differential equations is a set of linear equations relating a group of functions to their derivatives. If a(t) is an n n matrix function that is. Section 10.3 deals.
What are the differential equations? Types of Differential Equations
We show how to convert a system of. As with linear systems, a homogeneous linear system of di erential equations is one in which b(t) = 0. If a(t) is an n n matrix function that is. In this section we will look at some of the basics of systems of differential equations. Section 10.2 discusses linear systems of differential.
Solved (2) Systems of Linear Differential Equations and
Section 10.3 deals with the basic theory of homogeneous. A system of linear differential equations is a set of linear equations relating a group of functions to their derivatives. We show how to convert a system of. Section 10.2 discusses linear systems of differential equations. If a(t) is an n n matrix function that is.
In This Section We Will Look At Some Of The Basics Of Systems Of Differential Equations.
We show how to convert a system of. Section 10.2 discusses linear systems of differential equations. Section 10.3 deals with the basic theory of homogeneous. As with linear systems, a homogeneous linear system of di erential equations is one in which b(t) = 0.
If A(T) Is An N N Matrix Function That Is.
A linear system takes the form x0 = a(t)x +b(t)y +e(t) y0 = c(t)x +d(t)y + f(t).(6.7) a homogeneous linear system results when e(t) = 0 and f(t) = 0. A system of linear differential equations is a set of linear equations relating a group of functions to their derivatives.