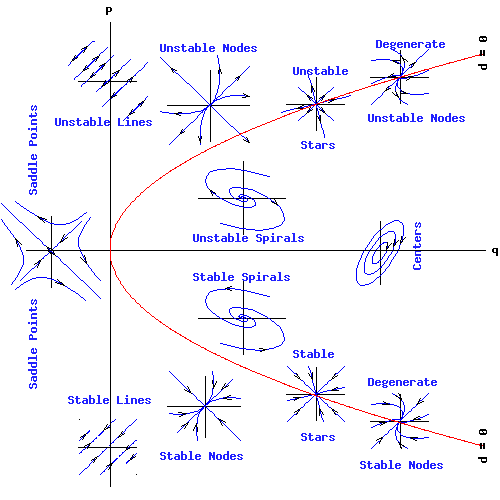
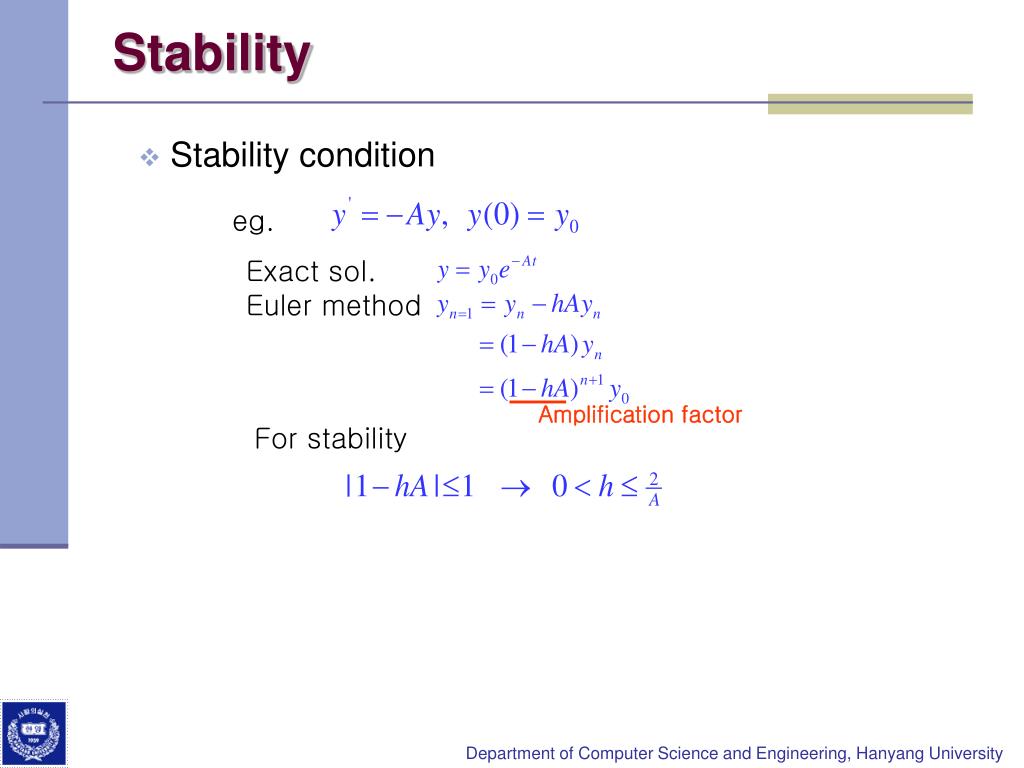
Stability Of A Differential Equation - We discuss properties of solutions of a first order two dimensional system, and stability theory for a special class of linear systems. Ai constants, t = time. Identifying stable and unstable equilibria of a differential equation by graphically solving the equation for nearby initial conditions.
We discuss properties of solutions of a first order two dimensional system, and stability theory for a special class of linear systems. Ai constants, t = time. Identifying stable and unstable equilibria of a differential equation by graphically solving the equation for nearby initial conditions.
Identifying stable and unstable equilibria of a differential equation by graphically solving the equation for nearby initial conditions. Ai constants, t = time. We discuss properties of solutions of a first order two dimensional system, and stability theory for a special class of linear systems.
Visualizing the stability of systems of ordinary differential equations
We discuss properties of solutions of a first order two dimensional system, and stability theory for a special class of linear systems. Identifying stable and unstable equilibria of a differential equation by graphically solving the equation for nearby initial conditions. Ai constants, t = time.
Stability for differential equation (11) Download Scientific Diagram
Ai constants, t = time. We discuss properties of solutions of a first order two dimensional system, and stability theory for a special class of linear systems. Identifying stable and unstable equilibria of a differential equation by graphically solving the equation for nearby initial conditions.
Egwald Mathematics Linear Algebra Systems of Linear Differential
We discuss properties of solutions of a first order two dimensional system, and stability theory for a special class of linear systems. Ai constants, t = time. Identifying stable and unstable equilibria of a differential equation by graphically solving the equation for nearby initial conditions.
Introduction of Differential Equation.pptx
We discuss properties of solutions of a first order two dimensional system, and stability theory for a special class of linear systems. Ai constants, t = time. Identifying stable and unstable equilibria of a differential equation by graphically solving the equation for nearby initial conditions.
(PDF) Stability and Consistency Analysis of Central Difference Scheme
Ai constants, t = time. Identifying stable and unstable equilibria of a differential equation by graphically solving the equation for nearby initial conditions. We discuss properties of solutions of a first order two dimensional system, and stability theory for a special class of linear systems.
PPT Numerical Analysis Differential Equation PowerPoint
Ai constants, t = time. We discuss properties of solutions of a first order two dimensional system, and stability theory for a special class of linear systems. Identifying stable and unstable equilibria of a differential equation by graphically solving the equation for nearby initial conditions.
dynamical systems Stability of fixed points for a differential
Identifying stable and unstable equilibria of a differential equation by graphically solving the equation for nearby initial conditions. We discuss properties of solutions of a first order two dimensional system, and stability theory for a special class of linear systems. Ai constants, t = time.
Stability results and existence for fractional differential equation
Identifying stable and unstable equilibria of a differential equation by graphically solving the equation for nearby initial conditions. Ai constants, t = time. We discuss properties of solutions of a first order two dimensional system, and stability theory for a special class of linear systems.
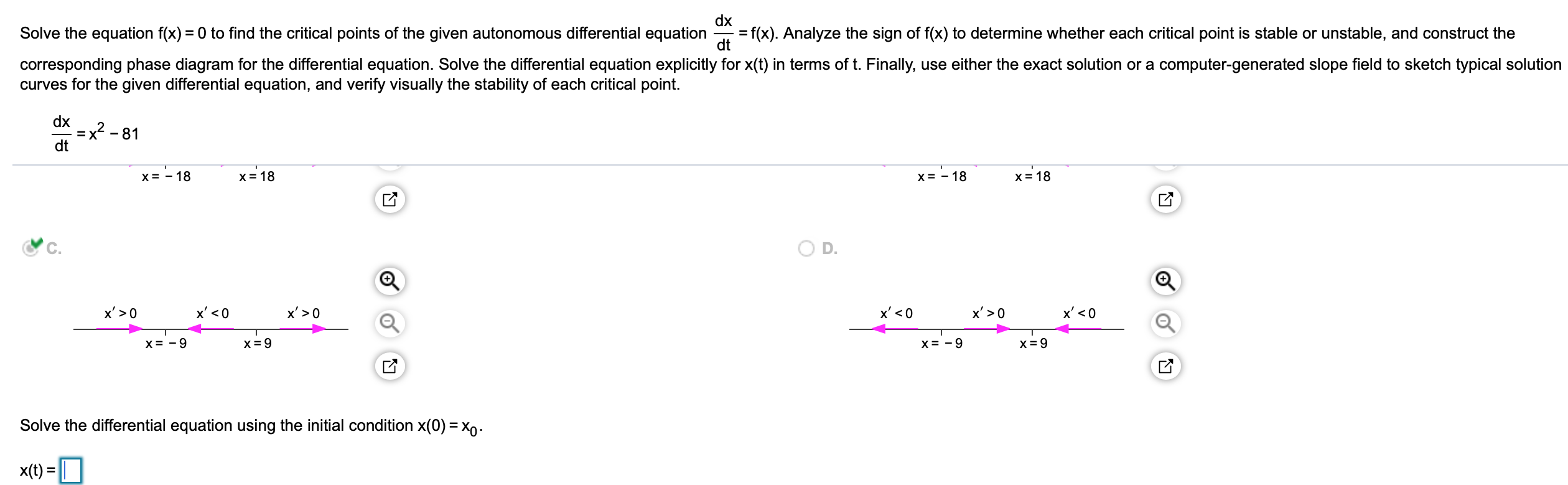
Solved dx Solve the equation f(x) = 0 to find the critical
Identifying stable and unstable equilibria of a differential equation by graphically solving the equation for nearby initial conditions. Ai constants, t = time. We discuss properties of solutions of a first order two dimensional system, and stability theory for a special class of linear systems.
(PDF) A note on exponential almost sure stability of stochastic
We discuss properties of solutions of a first order two dimensional system, and stability theory for a special class of linear systems. Identifying stable and unstable equilibria of a differential equation by graphically solving the equation for nearby initial conditions. Ai constants, t = time.
We Discuss Properties Of Solutions Of A First Order Two Dimensional System, And Stability Theory For A Special Class Of Linear Systems.
Ai constants, t = time. Identifying stable and unstable equilibria of a differential equation by graphically solving the equation for nearby initial conditions.