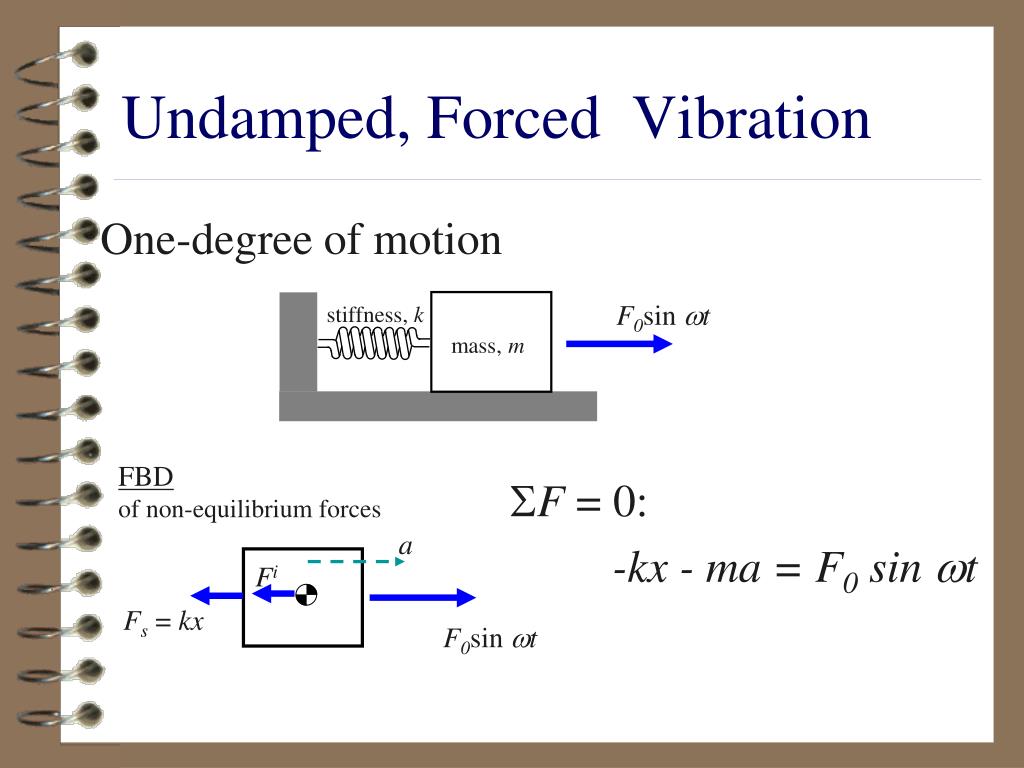
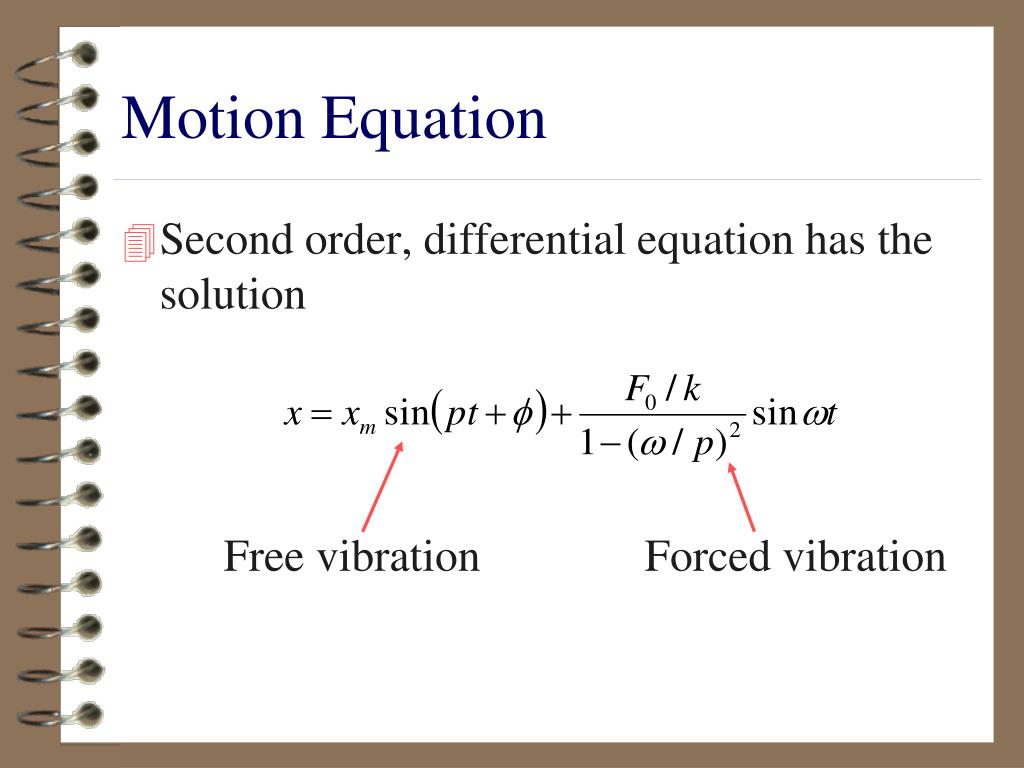
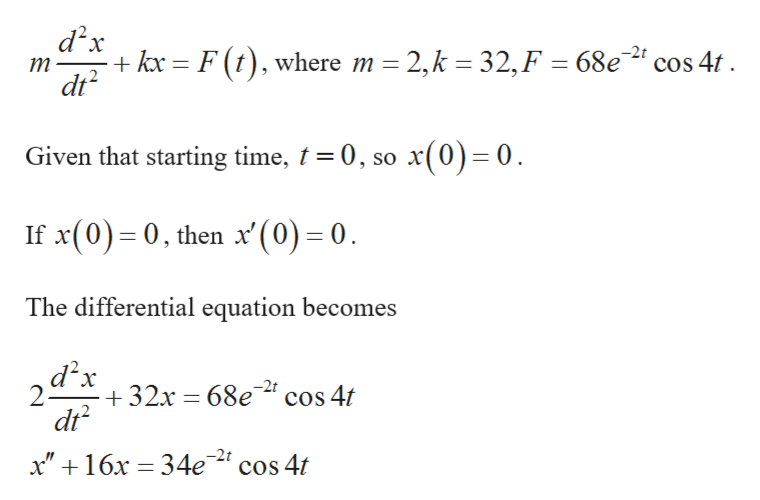Mechanical Vibrations Differential Equations - Simple mechanical vibrations satisfy the following differential equation: If the forcing function (𝑡) is not equals to zero, eq. By elementary principles we find li′ + ri + q c = e l i ′ + r i + q c = e. Mu′′(t) + γu′(t) + ku(t) = fexternal ,. Next we are also going to be using the following equations:
Simple mechanical vibrations satisfy the following differential equation: By elementary principles we find li′ + ri + q c = e l i ′ + r i + q c = e. Mu′′(t) + γu′(t) + ku(t) = fexternal ,. If the forcing function (𝑡) is not equals to zero, eq. Next we are also going to be using the following equations:
Next we are also going to be using the following equations: If the forcing function (𝑡) is not equals to zero, eq. Simple mechanical vibrations satisfy the following differential equation: By elementary principles we find li′ + ri + q c = e l i ′ + r i + q c = e. Mu′′(t) + γu′(t) + ku(t) = fexternal ,.
Day 24 MATH241 (Differential Equations) CH 3.7 Mechanical and
By elementary principles we find li′ + ri + q c = e l i ′ + r i + q c = e. Next we are also going to be using the following equations: Simple mechanical vibrations satisfy the following differential equation: If the forcing function (𝑡) is not equals to zero, eq. Mu′′(t) + γu′(t) + ku(t) =.
SOLVED 'This question is on mechanical vibrations in differential
Next we are also going to be using the following equations: Simple mechanical vibrations satisfy the following differential equation: By elementary principles we find li′ + ri + q c = e l i ′ + r i + q c = e. Mu′′(t) + γu′(t) + ku(t) = fexternal ,. If the forcing function (𝑡) is not equals to.
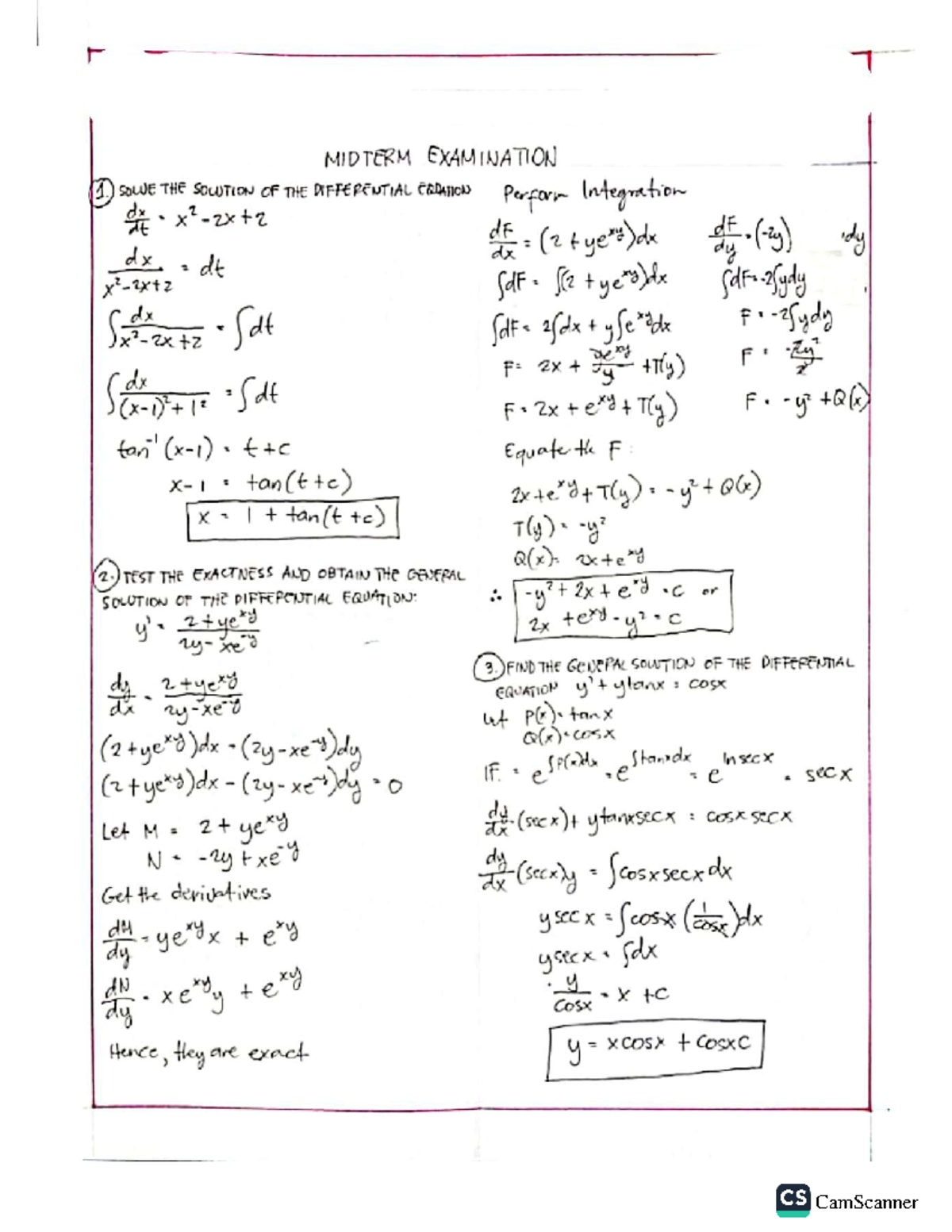
Differential Equations Midterm Examination Mechanical Engineering
Next we are also going to be using the following equations: By elementary principles we find li′ + ri + q c = e l i ′ + r i + q c = e. Mu′′(t) + γu′(t) + ku(t) = fexternal ,. If the forcing function (𝑡) is not equals to zero, eq. Simple mechanical vibrations satisfy the following.
Mechanical Engineering Mechanical Vibrations Multi Degree of Freedom
If the forcing function (𝑡) is not equals to zero, eq. Simple mechanical vibrations satisfy the following differential equation: Mu′′(t) + γu′(t) + ku(t) = fexternal ,. Next we are also going to be using the following equations: By elementary principles we find li′ + ri + q c = e l i ′ + r i + q c.
PPT Mechanical Vibrations PowerPoint Presentation, free download ID
Mu′′(t) + γu′(t) + ku(t) = fexternal ,. If the forcing function (𝑡) is not equals to zero, eq. Next we are also going to be using the following equations: By elementary principles we find li′ + ri + q c = e l i ′ + r i + q c = e. Simple mechanical vibrations satisfy the following.
Pauls Online Notes _ Differential Equations Mechanical Vibrations
If the forcing function (𝑡) is not equals to zero, eq. Mu′′(t) + γu′(t) + ku(t) = fexternal ,. By elementary principles we find li′ + ri + q c = e l i ′ + r i + q c = e. Simple mechanical vibrations satisfy the following differential equation: Next we are also going to be using the.
1/3 Mechanical Vibrations — Mnemozine
Next we are also going to be using the following equations: Mu′′(t) + γu′(t) + ku(t) = fexternal ,. By elementary principles we find li′ + ri + q c = e l i ′ + r i + q c = e. Simple mechanical vibrations satisfy the following differential equation: If the forcing function (𝑡) is not equals to.
PPT Mechanical Vibrations PowerPoint Presentation, free download ID
Next we are also going to be using the following equations: Simple mechanical vibrations satisfy the following differential equation: Mu′′(t) + γu′(t) + ku(t) = fexternal ,. If the forcing function (𝑡) is not equals to zero, eq. By elementary principles we find li′ + ri + q c = e l i ′ + r i + q c.
Forced Vibrations Notes 2018 PDF Damping Ordinary Differential
Simple mechanical vibrations satisfy the following differential equation: Next we are also going to be using the following equations: If the forcing function (𝑡) is not equals to zero, eq. By elementary principles we find li′ + ri + q c = e l i ′ + r i + q c = e. Mu′′(t) + γu′(t) + ku(t) =.
Answered Mechanincal Vibrations (Differential… bartleby
By elementary principles we find li′ + ri + q c = e l i ′ + r i + q c = e. Simple mechanical vibrations satisfy the following differential equation: Next we are also going to be using the following equations: Mu′′(t) + γu′(t) + ku(t) = fexternal ,. If the forcing function (𝑡) is not equals to.
Simple Mechanical Vibrations Satisfy The Following Differential Equation:
Next we are also going to be using the following equations: If the forcing function (𝑡) is not equals to zero, eq. By elementary principles we find li′ + ri + q c = e l i ′ + r i + q c = e. Mu′′(t) + γu′(t) + ku(t) = fexternal ,.