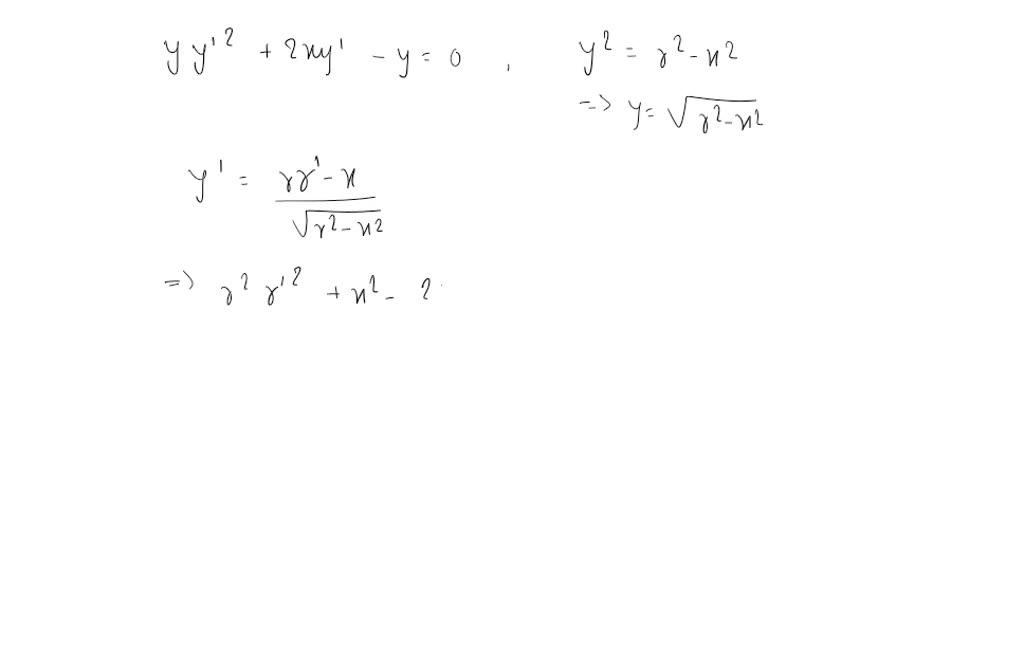How To Prove A Differential Equation Is Homogeneous - A first‐order differential equation is said to be homogeneous if m( x,y) and n( x,y) are both. A differential equation can be homogeneous in either of two respects. The best and the simplest test for checking the homogeneity of a differential.
The best and the simplest test for checking the homogeneity of a differential. A first‐order differential equation is said to be homogeneous if m( x,y) and n( x,y) are both. A differential equation can be homogeneous in either of two respects.
A differential equation can be homogeneous in either of two respects. The best and the simplest test for checking the homogeneity of a differential. A first‐order differential equation is said to be homogeneous if m( x,y) and n( x,y) are both.
SOLUTION Chapter 4 homogeneous differential equation Studypool
A differential equation can be homogeneous in either of two respects. A first‐order differential equation is said to be homogeneous if m( x,y) and n( x,y) are both. The best and the simplest test for checking the homogeneity of a differential.
[Solved] Solve the HOMOGENEOUS differential equation in step by step
A differential equation can be homogeneous in either of two respects. A first‐order differential equation is said to be homogeneous if m( x,y) and n( x,y) are both. The best and the simplest test for checking the homogeneity of a differential.
Solution of Homogeneous Linear Differential equation Yawin
A first‐order differential equation is said to be homogeneous if m( x,y) and n( x,y) are both. A differential equation can be homogeneous in either of two respects. The best and the simplest test for checking the homogeneity of a differential.
SOLUTION Homogeneous differential equation Studypool
A differential equation can be homogeneous in either of two respects. The best and the simplest test for checking the homogeneity of a differential. A first‐order differential equation is said to be homogeneous if m( x,y) and n( x,y) are both.
3. Differential Equation Homogeneous Differential Lecture.2024.pptx
The best and the simplest test for checking the homogeneity of a differential. A first‐order differential equation is said to be homogeneous if m( x,y) and n( x,y) are both. A differential equation can be homogeneous in either of two respects.
SOLVED Prove that if a homogeneous firstorder differential equation
A differential equation can be homogeneous in either of two respects. A first‐order differential equation is said to be homogeneous if m( x,y) and n( x,y) are both. The best and the simplest test for checking the homogeneity of a differential.
Homogeneous Differential Equation Know types, Steps to solve
A first‐order differential equation is said to be homogeneous if m( x,y) and n( x,y) are both. A differential equation can be homogeneous in either of two respects. The best and the simplest test for checking the homogeneity of a differential.
3. Differential Equation Homogeneous Differential Lecture.2024.pptx
A first‐order differential equation is said to be homogeneous if m( x,y) and n( x,y) are both. A differential equation can be homogeneous in either of two respects. The best and the simplest test for checking the homogeneity of a differential.
please derive the homogeneous differential equation of this question
A differential equation can be homogeneous in either of two respects. The best and the simplest test for checking the homogeneity of a differential. A first‐order differential equation is said to be homogeneous if m( x,y) and n( x,y) are both.
SOLUTION Homogeneous differential equation Studypool
A first‐order differential equation is said to be homogeneous if m( x,y) and n( x,y) are both. A differential equation can be homogeneous in either of two respects. The best and the simplest test for checking the homogeneity of a differential.
A First‐Order Differential Equation Is Said To Be Homogeneous If M( X,Y) And N( X,Y) Are Both.
A differential equation can be homogeneous in either of two respects. The best and the simplest test for checking the homogeneity of a differential.