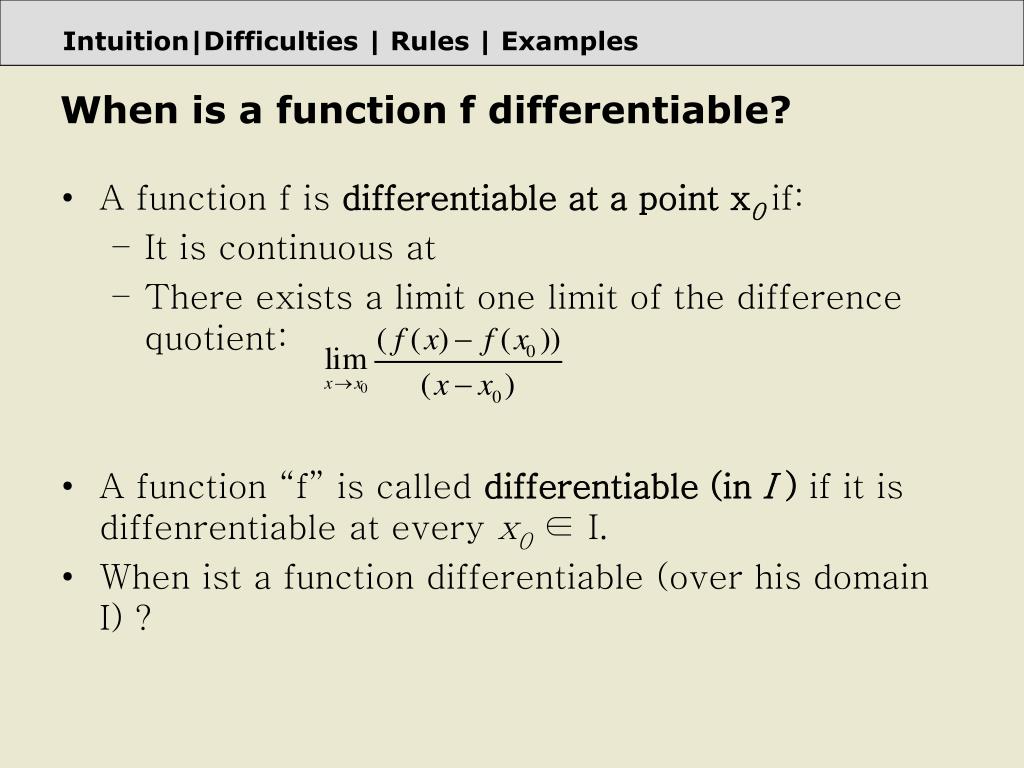
How To Know If Function Is Differentiable - Lim h→0 [(f(x + h) − f(x)) / h]. If the limit exists for a particular x,. We can determine if a function is differentiable at a point by using the formula:
We can determine if a function is differentiable at a point by using the formula: Lim h→0 [(f(x + h) − f(x)) / h]. If the limit exists for a particular x,.
If the limit exists for a particular x,. Lim h→0 [(f(x + h) − f(x)) / h]. We can determine if a function is differentiable at a point by using the formula:
calculus Continuous,Discontinuous ,Differential and non
Lim h→0 [(f(x + h) − f(x)) / h]. If the limit exists for a particular x,. We can determine if a function is differentiable at a point by using the formula:
Solved We know that a differentiable function is always
We can determine if a function is differentiable at a point by using the formula: Lim h→0 [(f(x + h) − f(x)) / h]. If the limit exists for a particular x,.
Is a Function Differentiable at a Hole
We can determine if a function is differentiable at a point by using the formula: Lim h→0 [(f(x + h) − f(x)) / h]. If the limit exists for a particular x,.
Differentiable function Wikiwand
We can determine if a function is differentiable at a point by using the formula: If the limit exists for a particular x,. Lim h→0 [(f(x + h) − f(x)) / h].
How to Know if a Function is Differentiable A Simple Guide
If the limit exists for a particular x,. We can determine if a function is differentiable at a point by using the formula: Lim h→0 [(f(x + h) − f(x)) / h].
When Is a Function Continuous but Not Differentiable Quant RL
Lim h→0 [(f(x + h) − f(x)) / h]. We can determine if a function is differentiable at a point by using the formula: If the limit exists for a particular x,.
Differentiable Cuemath
We can determine if a function is differentiable at a point by using the formula: Lim h→0 [(f(x + h) − f(x)) / h]. If the limit exists for a particular x,.
Is a Function Differentiable at a Hole
Lim h→0 [(f(x + h) − f(x)) / h]. If the limit exists for a particular x,. We can determine if a function is differentiable at a point by using the formula:
PPT Differentiable Functions PowerPoint Presentation, free download
Lim h→0 [(f(x + h) − f(x)) / h]. We can determine if a function is differentiable at a point by using the formula: If the limit exists for a particular x,.
Differentiable Function Meaning, Formulas and Examples Outlier
We can determine if a function is differentiable at a point by using the formula: Lim h→0 [(f(x + h) − f(x)) / h]. If the limit exists for a particular x,.
We Can Determine If A Function Is Differentiable At A Point By Using The Formula:
Lim h→0 [(f(x + h) − f(x)) / h]. If the limit exists for a particular x,.