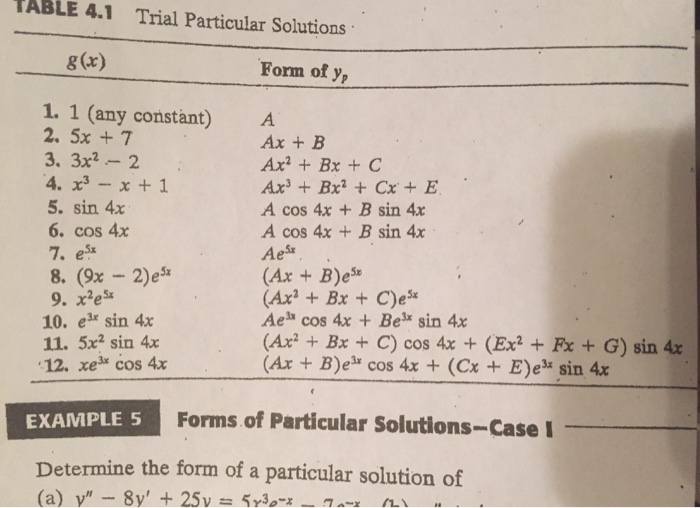
Homogeneous Vs Nonhomogeneous Differential Equations - The simplest test of homogeneity, and definition at the same time, not only for differential equations, is the following: Solve a nonhomogeneous differential equation by the. (1) and (2) are of the form $$ \mathcal{d} u = 0 $$ where $\mathcal d$ is a differential operator. Write the general solution to a nonhomogeneous differential equation. Thus, these differential equations are. A differential equation of the form f(x,y)dy = g(x,y)dx is said to be homogeneous differential equation if the degree of f(x,y) and g(x, y) is.
Write the general solution to a nonhomogeneous differential equation. Solve a nonhomogeneous differential equation by the. (1) and (2) are of the form $$ \mathcal{d} u = 0 $$ where $\mathcal d$ is a differential operator. The simplest test of homogeneity, and definition at the same time, not only for differential equations, is the following: Thus, these differential equations are. A differential equation of the form f(x,y)dy = g(x,y)dx is said to be homogeneous differential equation if the degree of f(x,y) and g(x, y) is.
Thus, these differential equations are. The simplest test of homogeneity, and definition at the same time, not only for differential equations, is the following: Write the general solution to a nonhomogeneous differential equation. A differential equation of the form f(x,y)dy = g(x,y)dx is said to be homogeneous differential equation if the degree of f(x,y) and g(x, y) is. (1) and (2) are of the form $$ \mathcal{d} u = 0 $$ where $\mathcal d$ is a differential operator. Solve a nonhomogeneous differential equation by the.
PPT Homogeneous Differential Equation NonHomogeneous Differential
Thus, these differential equations are. (1) and (2) are of the form $$ \mathcal{d} u = 0 $$ where $\mathcal d$ is a differential operator. A differential equation of the form f(x,y)dy = g(x,y)dx is said to be homogeneous differential equation if the degree of f(x,y) and g(x, y) is. Solve a nonhomogeneous differential equation by the. The simplest test.
Particular Solution of NonHomogeneous Differential Equations Mr
Write the general solution to a nonhomogeneous differential equation. The simplest test of homogeneity, and definition at the same time, not only for differential equations, is the following: (1) and (2) are of the form $$ \mathcal{d} u = 0 $$ where $\mathcal d$ is a differential operator. Solve a nonhomogeneous differential equation by the. A differential equation of the.
[Differential Equations] NonHomogeneous Equation Solution r/learnmath
Thus, these differential equations are. A differential equation of the form f(x,y)dy = g(x,y)dx is said to be homogeneous differential equation if the degree of f(x,y) and g(x, y) is. (1) and (2) are of the form $$ \mathcal{d} u = 0 $$ where $\mathcal d$ is a differential operator. Write the general solution to a nonhomogeneous differential equation. The.
[Solved] A nonhomogeneous differential equation, a complementary
Thus, these differential equations are. A differential equation of the form f(x,y)dy = g(x,y)dx is said to be homogeneous differential equation if the degree of f(x,y) and g(x, y) is. The simplest test of homogeneity, and definition at the same time, not only for differential equations, is the following: Write the general solution to a nonhomogeneous differential equation. Solve a.
Solved Problem type Differential Equations Nonhomogeneous
(1) and (2) are of the form $$ \mathcal{d} u = 0 $$ where $\mathcal d$ is a differential operator. Write the general solution to a nonhomogeneous differential equation. A differential equation of the form f(x,y)dy = g(x,y)dx is said to be homogeneous differential equation if the degree of f(x,y) and g(x, y) is. The simplest test of homogeneity, and.
SOLUTION SOLUTION NonHomogeneous Linear Differential Equations with
Thus, these differential equations are. The simplest test of homogeneity, and definition at the same time, not only for differential equations, is the following: (1) and (2) are of the form $$ \mathcal{d} u = 0 $$ where $\mathcal d$ is a differential operator. Solve a nonhomogeneous differential equation by the. A differential equation of the form f(x,y)dy = g(x,y)dx.
(PDF) Some Notes on the Solutions of non Homogeneous Differential Equations
The simplest test of homogeneity, and definition at the same time, not only for differential equations, is the following: (1) and (2) are of the form $$ \mathcal{d} u = 0 $$ where $\mathcal d$ is a differential operator. Thus, these differential equations are. A differential equation of the form f(x,y)dy = g(x,y)dx is said to be homogeneous differential equation.
How To Identify NonHomogeneous Differential Equations Fast
(1) and (2) are of the form $$ \mathcal{d} u = 0 $$ where $\mathcal d$ is a differential operator. A differential equation of the form f(x,y)dy = g(x,y)dx is said to be homogeneous differential equation if the degree of f(x,y) and g(x, y) is. The simplest test of homogeneity, and definition at the same time, not only for differential.
Differential Equations Lecture NonHomogeneous Linear Differential E…
Solve a nonhomogeneous differential equation by the. Thus, these differential equations are. The simplest test of homogeneity, and definition at the same time, not only for differential equations, is the following: (1) and (2) are of the form $$ \mathcal{d} u = 0 $$ where $\mathcal d$ is a differential operator. A differential equation of the form f(x,y)dy = g(x,y)dx.
How To Identify NonHomogeneous Differential Equations Fast
The simplest test of homogeneity, and definition at the same time, not only for differential equations, is the following: Solve a nonhomogeneous differential equation by the. Thus, these differential equations are. Write the general solution to a nonhomogeneous differential equation. (1) and (2) are of the form $$ \mathcal{d} u = 0 $$ where $\mathcal d$ is a differential operator.
(1) And (2) Are Of The Form $$ \Mathcal{D} U = 0 $$ Where $\Mathcal D$ Is A Differential Operator.
Solve a nonhomogeneous differential equation by the. Thus, these differential equations are. The simplest test of homogeneity, and definition at the same time, not only for differential equations, is the following: A differential equation of the form f(x,y)dy = g(x,y)dx is said to be homogeneous differential equation if the degree of f(x,y) and g(x, y) is.


![[Differential Equations] NonHomogeneous Equation Solution r/learnmath](https://i.imgur.com/6lFKSuI.png)