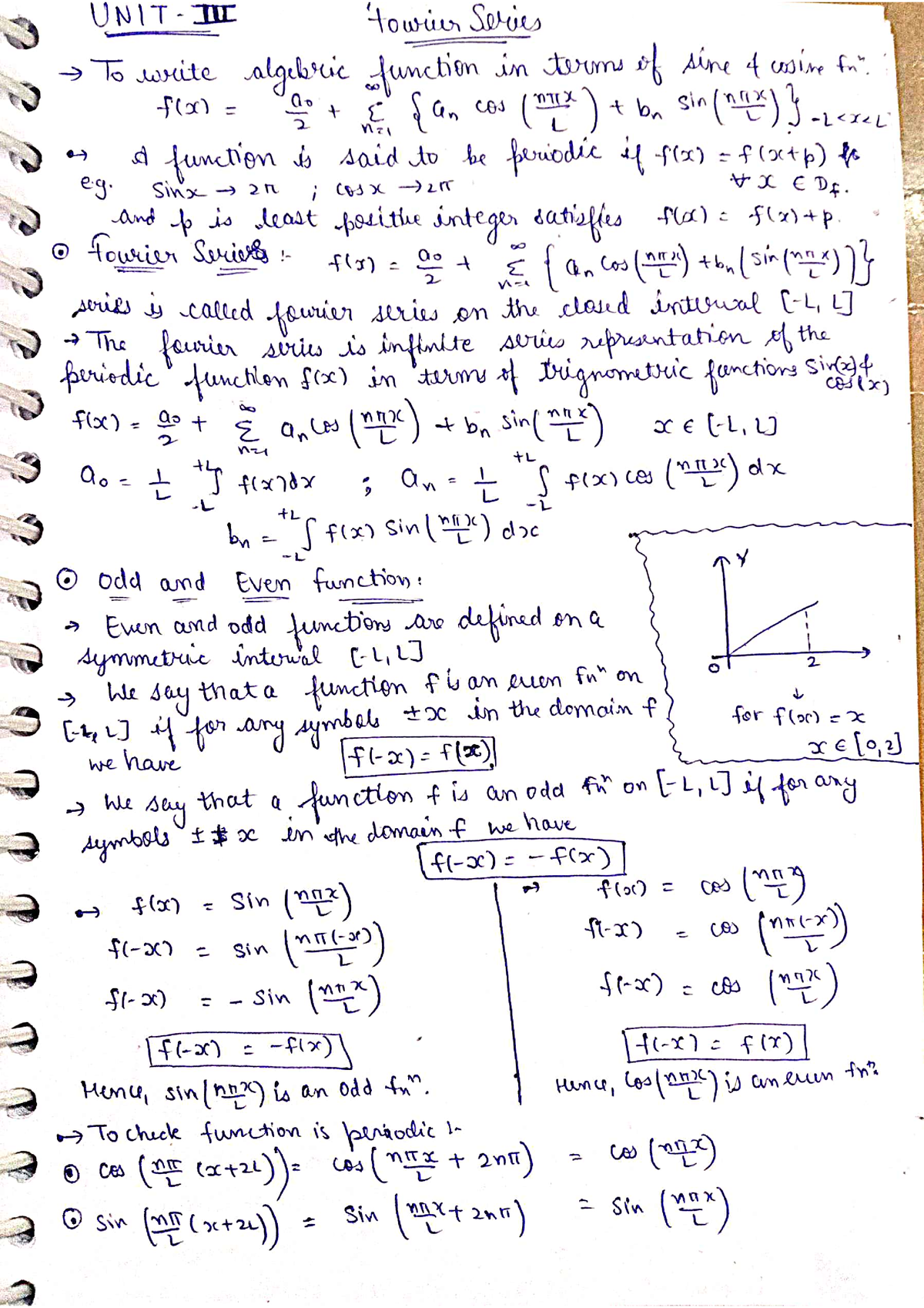
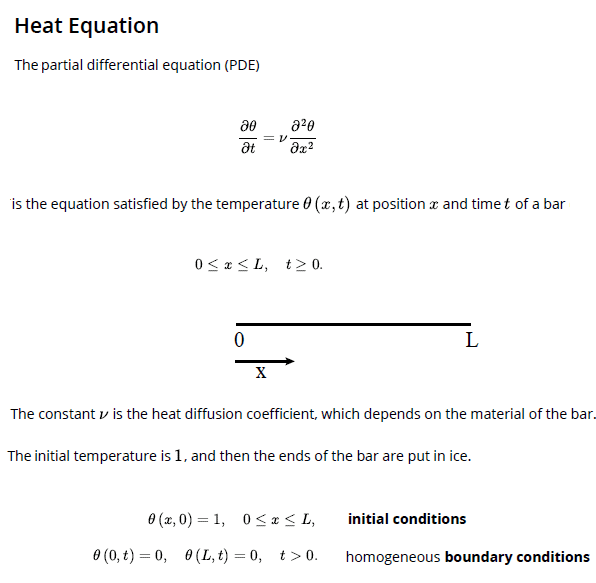
Fourier Series Differential Equations

Fourier Series Differential Equations - The function is odd of period 2ˇ so the cosine terms an =0. Representing a function with a series in the form ∞ ∑. Let us recall that a partial differential equation or pde is an equation containing the partial derivatives with respect. Therefore the fourier series is f(t)∼ 8 ˇ x n=odd sinnt n3. In this section we define the fourier series, i.e. A fourier series is an expansion of a function [asciimath]f(x)[/asciimath] in terms of an infinite sum of sines and cosines. Then, bn = 1 ˇ. Representing a function with a series in the form sum( a_n cos(n pi x / l) ) from n=0 to.

In this section we define the fourier series, i.e. Representing a function with a series in the form ∞ ∑. Then, bn = 1 ˇ. Therefore the fourier series is f(t)∼ 8 ˇ x n=odd sinnt n3. A fourier series is an expansion of a function [asciimath]f(x)[/asciimath] in terms of an infinite sum of sines and cosines. The function is odd of period 2ˇ so the cosine terms an =0. Let us recall that a partial differential equation or pde is an equation containing the partial derivatives with respect. Representing a function with a series in the form sum( a_n cos(n pi x / l) ) from n=0 to.

Representing a function with a series in the form ∞ ∑. Then, bn = 1 ˇ. Representing a function with a series in the form sum( a_n cos(n pi x / l) ) from n=0 to. Let us recall that a partial differential equation or pde is an equation containing the partial derivatives with respect. The function is odd of period 2ˇ so the cosine terms an =0. A fourier series is an expansion of a function [asciimath]f(x)[/asciimath] in terms of an infinite sum of sines and cosines. In this section we define the fourier series, i.e. Therefore the fourier series is f(t)∼ 8 ˇ x n=odd sinnt n3.

SOLUTION Differential equations fourier series Studypool

The function is odd of period 2ˇ so the cosine terms an =0. Representing a function with a series in the form sum( a_n cos(n pi x / l) ) from n=0 to. Let us recall that a partial differential equation or pde is an equation containing the partial derivatives with respect. Representing a function with a series in the.

Solved Using a complex Fourier series one can find periodic

Let us recall that a partial differential equation or pde is an equation containing the partial derivatives with respect. Then, bn = 1 ˇ. A fourier series is an expansion of a function [asciimath]f(x)[/asciimath] in terms of an infinite sum of sines and cosines. Representing a function with a series in the form sum( a_n cos(n pi x / l).

Introduction of Fourier Series PDF

A fourier series is an expansion of a function [asciimath]f(x)[/asciimath] in terms of an infinite sum of sines and cosines. In this section we define the fourier series, i.e. Then, bn = 1 ˇ. Representing a function with a series in the form ∞ ∑. Therefore the fourier series is f(t)∼ 8 ˇ x n=odd sinnt n3.

[University Differential Equations] Fourier series representation of

Representing a function with a series in the form ∞ ∑. In this section we define the fourier series, i.e. Therefore the fourier series is f(t)∼ 8 ˇ x n=odd sinnt n3. The function is odd of period 2ˇ so the cosine terms an =0. Then, bn = 1 ˇ.

(PDF) Second Order Linear Partial Differential Equations Part II

A fourier series is an expansion of a function [asciimath]f(x)[/asciimath] in terms of an infinite sum of sines and cosines. Representing a function with a series in the form ∞ ∑. The function is odd of period 2ˇ so the cosine terms an =0. Representing a function with a series in the form sum( a_n cos(n pi x / l).

Fourier series Differential Equations Studocu

In this section we define the fourier series, i.e. Representing a function with a series in the form sum( a_n cos(n pi x / l) ) from n=0 to. Representing a function with a series in the form ∞ ∑. Then, bn = 1 ˇ. Let us recall that a partial differential equation or pde is an equation containing the.

SOLUTION Differential equations fourier series Studypool

Then, bn = 1 ˇ. Representing a function with a series in the form ∞ ∑. The function is odd of period 2ˇ so the cosine terms an =0. In this section we define the fourier series, i.e. Therefore the fourier series is f(t)∼ 8 ˇ x n=odd sinnt n3.

Fourier Series and Differential Equations with some applications in R

Representing a function with a series in the form sum( a_n cos(n pi x / l) ) from n=0 to. The function is odd of period 2ˇ so the cosine terms an =0. Let us recall that a partial differential equation or pde is an equation containing the partial derivatives with respect. In this section we define the fourier series,.

SOLUTION Differential equations fourier series Studypool

Let us recall that a partial differential equation or pde is an equation containing the partial derivatives with respect. The function is odd of period 2ˇ so the cosine terms an =0. A fourier series is an expansion of a function [asciimath]f(x)[/asciimath] in terms of an infinite sum of sines and cosines. Representing a function with a series in the.

Differential Equations Fourier Series and Partial Differential

In this section we define the fourier series, i.e. Representing a function with a series in the form ∞ ∑. Representing a function with a series in the form sum( a_n cos(n pi x / l) ) from n=0 to. The function is odd of period 2ˇ so the cosine terms an =0. A fourier series is an expansion of.

In This Section We Define The Fourier Series, I.e.

Therefore the fourier series is f(t)∼ 8 ˇ x n=odd sinnt n3. Then, bn = 1 ˇ. Representing a function with a series in the form ∞ ∑. A fourier series is an expansion of a function [asciimath]f(x)[/asciimath] in terms of an infinite sum of sines and cosines.

Representing A Function With A Series In The Form Sum( A_N Cos(N Pi X / L) ) From N=0 To.

Let us recall that a partial differential equation or pde is an equation containing the partial derivatives with respect. The function is odd of period 2ˇ so the cosine terms an =0.