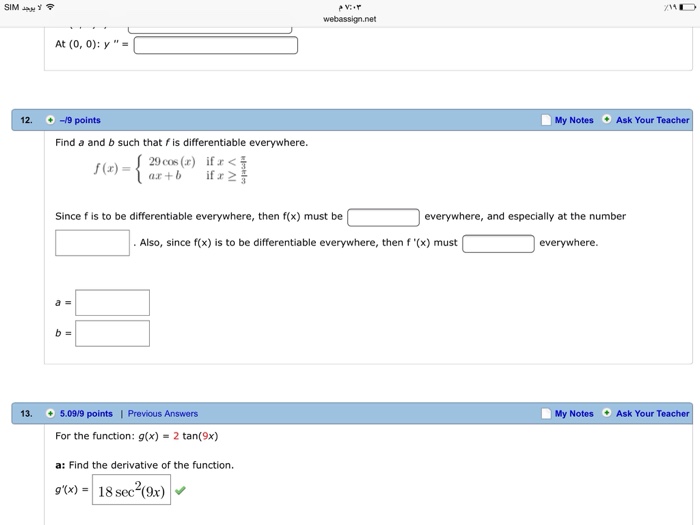
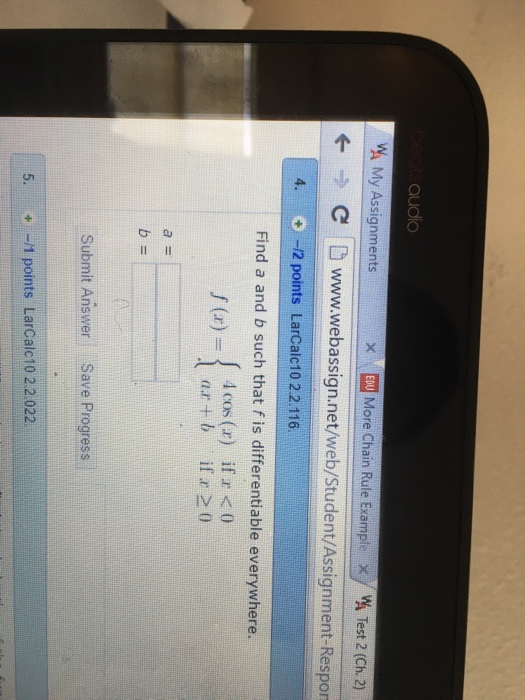
Find A And B Such That F Is Differentiable Everywhere - There are 4 steps to solve this one. The values of a and b that make the function f differentiable everywhere are: $$(x^2+b)' = 2x+b$$ the correct way to get the value of $b$: (a) find the values of a and b such that f(x) is differentiable everywhere and compute f′(x). Find a and b such that f is differentiable everywhere. If and only if lim x → c − f (x) = lim x → c + f (x) =. F '(x) = acos(ax) then plug in x = 0 to get: Therefore, f(x) = 4 cos(x) for x < 0, and. F '(x) = acos(a(0)) = a•1 = a. By equating the two parts of the piecewise function at the.
Function $f(x)$ must be continuous at $x=2$. The values of a and b that make the function f differentiable everywhere are: If and only if lim x → c − f (x) = lim x → c + f (x) =. Therefore, f(x) = 4 cos(x) for x < 0, and. (a) find the values of a and b such that f(x) is differentiable everywhere and compute f′(x). To ensure that the function f(x) is. F '(x) = acos(ax) then plug in x = 0 to get: F '(x) = acos(a(0)) = a•1 = a. F(x) = sin(ax) + b. Find a and b such that f is differentiable everywhere.
By equating the two parts of the piecewise function at the. To make f differentiable everywhere, we set a = 0 and b can be any real number. There are 4 steps to solve this one. Find all values of and that make the following. For f (x) to be differentiable everywhere, it must first be continuous everywhere. (b) is the function f ′ (x) differentiable. To ensure that the function f(x) is. (a) find the values of a and b such that f(x) is differentiable everywhere and compute f′(x). F '(x) = acos(ax) then plug in x = 0 to get: Therefore, f(x) = 4 cos(x) for x < 0, and.
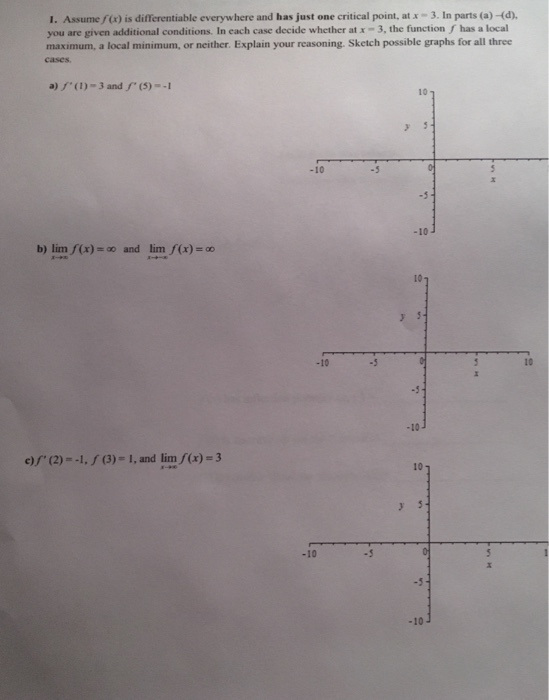
Solved 1. Assume f() is differentiable everywhere and has
(b) is the function f ′ (x) differentiable. If and only if lim x → c − f (x) = lim x → c + f (x) =. (a) find the values of a and b such that f(x) is differentiable everywhere and compute f′(x). $$(x^2+b)' = 2x+b$$ the correct way to get the value of $b$: F(x) = sin(ax).
Solved Find a and b such that f is differentiable
By equating the two parts of the piecewise function at the. Function $f(x)$ must be continuous at $x=2$. (b) is the function f ′ (x) differentiable. To make f differentiable everywhere, we set a = 0 and b can be any real number. Find all values of and that make the following.
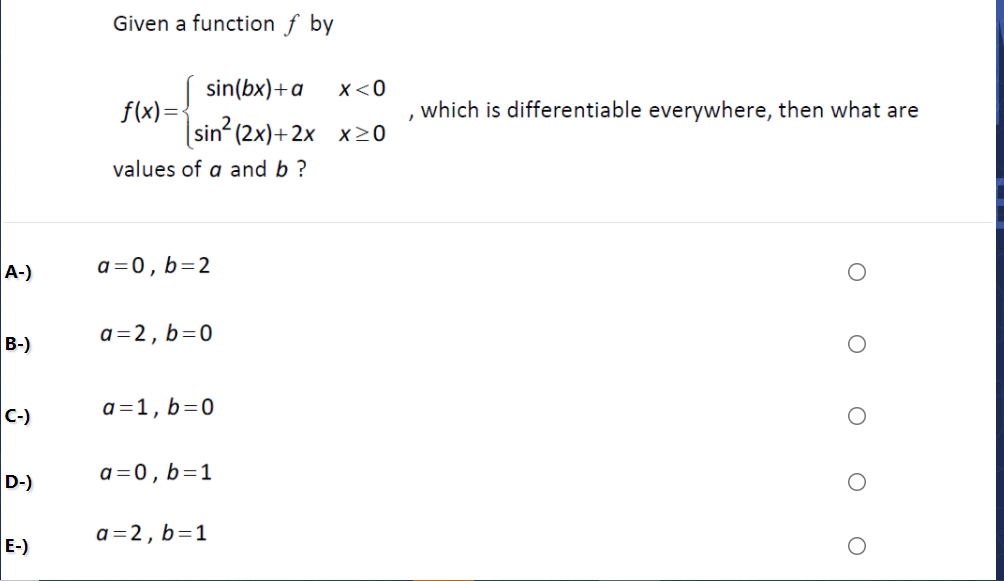
Solved Given a function f by which is differentiable
F '(x) = acos(ax) then plug in x = 0 to get: (b) is the function f ′ (x) differentiable. Function $f(x)$ must be continuous at $x=2$. The values of a and b that make the function f differentiable everywhere are: Find a and b such that f is differentiable everywhere.
Let f[0,1]→R is a differentiable function such that f(0) = 0 and f(x
To ensure that the function f(x) is. F '(x) = acos(a(0)) = a•1 = a. If and only if lim x → c − f (x) = lim x → c + f (x) =. Therefore, f(x) = 4 cos(x) for x < 0, and. Find all values of and that make the following.
Solved Find the value of m which makes f (x) differentiable
(a) find the values of a and b such that f(x) is differentiable everywhere and compute f′(x). $$(x^2+b)' = 2x+b$$ the correct way to get the value of $b$: Function $f(x)$ must be continuous at $x=2$. Find all values of and that make the following. If and only if lim x → c − f (x) = lim x →.
Solved Find the Values of m and b that make f(x)
Find all values of and that make the following. F(x) = sin(ax) + b. To ensure that the function f(x) is. For f (x) to be differentiable everywhere, it must first be continuous everywhere. The values of a and b that make the function f differentiable everywhere are:
Solved Find a and b such that f is differentiable
By equating the two parts of the piecewise function at the. Therefore, f(x) = 4 cos(x) for x < 0, and. (a) find the values of a and b such that f(x) is differentiable everywhere and compute f′(x). There are 4 steps to solve this one. To make f differentiable everywhere, we set a = 0 and b can be.
Let f and g be differentiable on [0 , 1] such that f(0)=2 , g(0)=0, f(1
There are 4 steps to solve this one. Therefore, f(x) = 4 cos(x) for x < 0, and. (b) is the function f ′ (x) differentiable. For f (x) to be differentiable everywhere, it must first be continuous everywhere. To make f differentiable everywhere, we set a = 0 and b can be any real number.
Solved Find a and b such that f is differentiable
(b) is the function f ′ (x) differentiable. To ensure that the function f(x) is. If and only if lim x → c − f (x) = lim x → c + f (x) =. (a) find the values of a and b such that f(x) is differentiable everywhere and compute f′(x). $$(x^2+b)' = 2x+b$$ the correct way to get.
If f x is a twice differentiable function such that f a =0, f b =2, f c
$$(x^2+b)' = 2x+b$$ the correct way to get the value of $b$: For f (x) to be differentiable everywhere, it must first be continuous everywhere. Find a and b such that f is differentiable everywhere. By equating the two parts of the piecewise function at the. (b) is the function f ′ (x) differentiable.
(A) Find The Values Of A And B Such That F(X) Is Differentiable Everywhere And Compute F′(X).
Therefore, f(x) = 4 cos(x) for x < 0, and. F '(x) = acos(ax) then plug in x = 0 to get: To ensure that the function f(x) is. By equating the two parts of the piecewise function at the.
Find All Values Of And That Make The Following.
The values of a and b that make the function f differentiable everywhere are: For f (x) to be differentiable everywhere, it must first be continuous everywhere. F '(x) = acos(a(0)) = a•1 = a. If and only if lim x → c − f (x) = lim x → c + f (x) =.
F(X) = Sin(Ax) + B.
Function $f(x)$ must be continuous at $x=2$. $$(x^2+b)' = 2x+b$$ the correct way to get the value of $b$: There are 4 steps to solve this one. Find a and b such that f is differentiable everywhere.
(B) Is The Function F ′ (X) Differentiable.
To make f differentiable everywhere, we set a = 0 and b can be any real number.

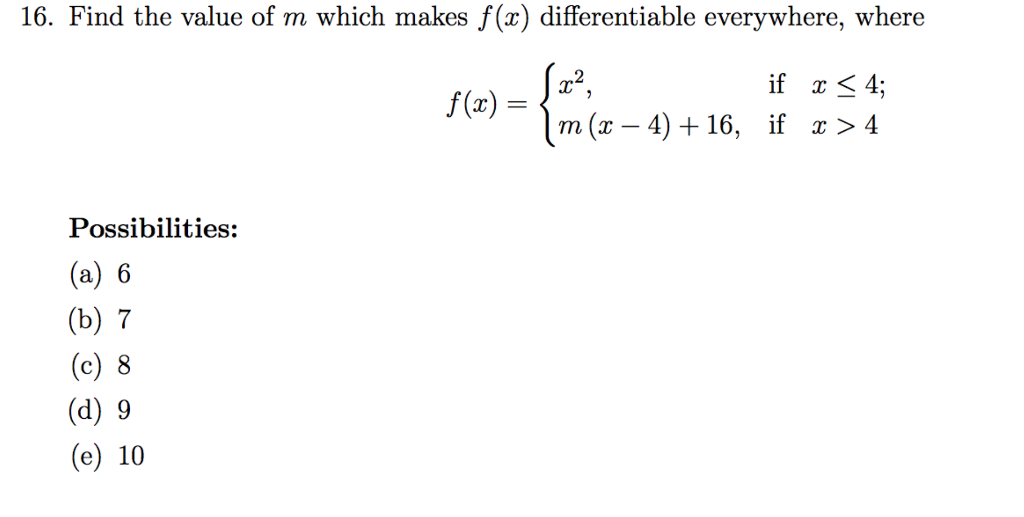

![Let f and g be differentiable on [0 , 1] such that f(0)=2 , g(0)=0, f(1](https://search-static.byjusweb.com/question-images/toppr_invalid/questions/1445190_712243_ans_46fd370c4aa44de4859d8fab8b50d829.jpeg)