Existence Theorem For Differential Equations - Then the differential equation (2) with initial con. Notes on the existence and uniqueness theorem for first order differential equations i. It guarantees that a solution exists on. (a) is an existence theorem. Let the function f(t,y) be continuous and satisfy the bound (3). It’s important to understand exactly what theorem 1.2.1 says.
Then the differential equation (2) with initial con. Notes on the existence and uniqueness theorem for first order differential equations i. (a) is an existence theorem. Let the function f(t,y) be continuous and satisfy the bound (3). It’s important to understand exactly what theorem 1.2.1 says. It guarantees that a solution exists on.
It guarantees that a solution exists on. (a) is an existence theorem. Then the differential equation (2) with initial con. Notes on the existence and uniqueness theorem for first order differential equations i. It’s important to understand exactly what theorem 1.2.1 says. Let the function f(t,y) be continuous and satisfy the bound (3).
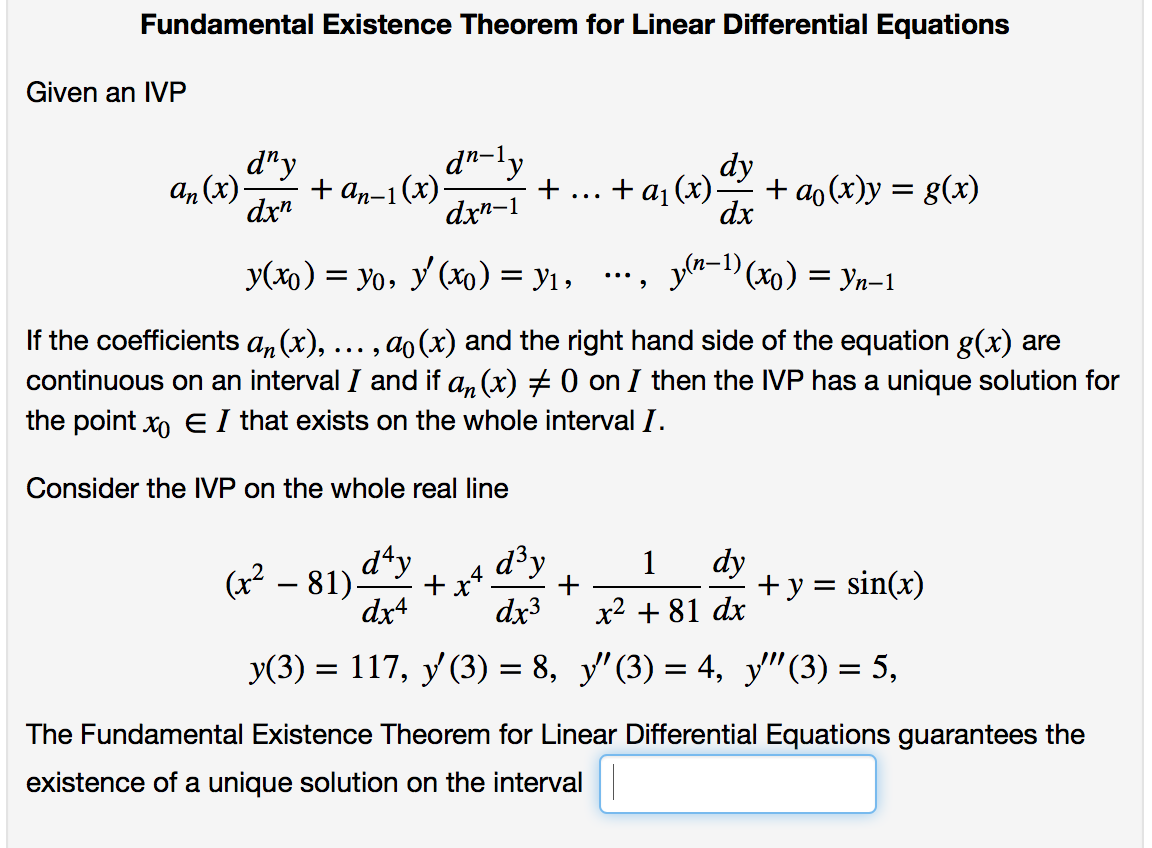
Solved The Fundamental Existence Theorem for Linear
Then the differential equation (2) with initial con. It guarantees that a solution exists on. (a) is an existence theorem. It’s important to understand exactly what theorem 1.2.1 says. Let the function f(t,y) be continuous and satisfy the bound (3).
ordinary differential equations Existence and Uniqueness Theorem
It’s important to understand exactly what theorem 1.2.1 says. (a) is an existence theorem. Let the function f(t,y) be continuous and satisfy the bound (3). Notes on the existence and uniqueness theorem for first order differential equations i. It guarantees that a solution exists on.
(PDF) EXISTENCE THEOREM FOR LINEAR NEUTRAL IMPULSIVE DIFFERENTIAL
Then the differential equation (2) with initial con. It guarantees that a solution exists on. It’s important to understand exactly what theorem 1.2.1 says. Notes on the existence and uniqueness theorem for first order differential equations i. (a) is an existence theorem.
Lesson 7 Existence And Uniqueness Theorem (Differential Equations
Let the function f(t,y) be continuous and satisfy the bound (3). It’s important to understand exactly what theorem 1.2.1 says. (a) is an existence theorem. Then the differential equation (2) with initial con. Notes on the existence and uniqueness theorem for first order differential equations i.
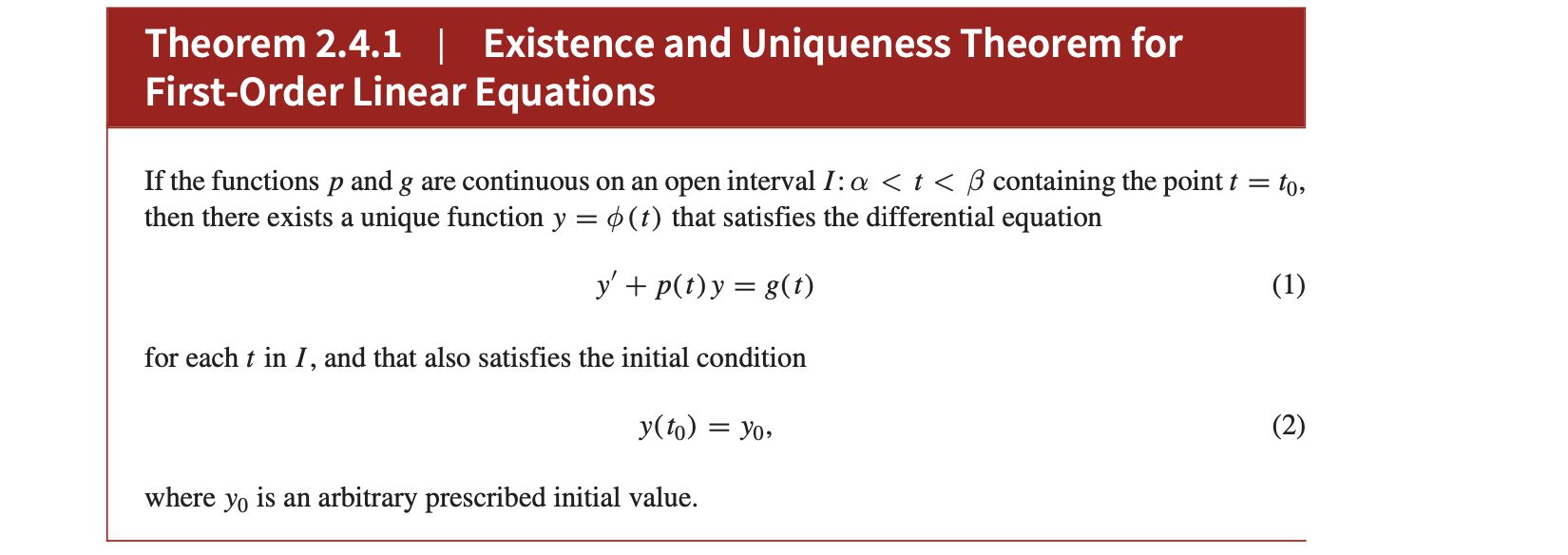
Solved Theorem 2.4.1 Existence and Uniqueness Theorem for
It guarantees that a solution exists on. (a) is an existence theorem. Then the differential equation (2) with initial con. It’s important to understand exactly what theorem 1.2.1 says. Let the function f(t,y) be continuous and satisfy the bound (3).
(PDF) New Proof for the Theorem of Existence and Uniqueness of a Class
Notes on the existence and uniqueness theorem for first order differential equations i. Then the differential equation (2) with initial con. It’s important to understand exactly what theorem 1.2.1 says. It guarantees that a solution exists on. (a) is an existence theorem.
(PDF) Existence results by Mönch's fixed point theorem for a tripled
Let the function f(t,y) be continuous and satisfy the bound (3). Then the differential equation (2) with initial con. It guarantees that a solution exists on. Notes on the existence and uniqueness theorem for first order differential equations i. It’s important to understand exactly what theorem 1.2.1 says.
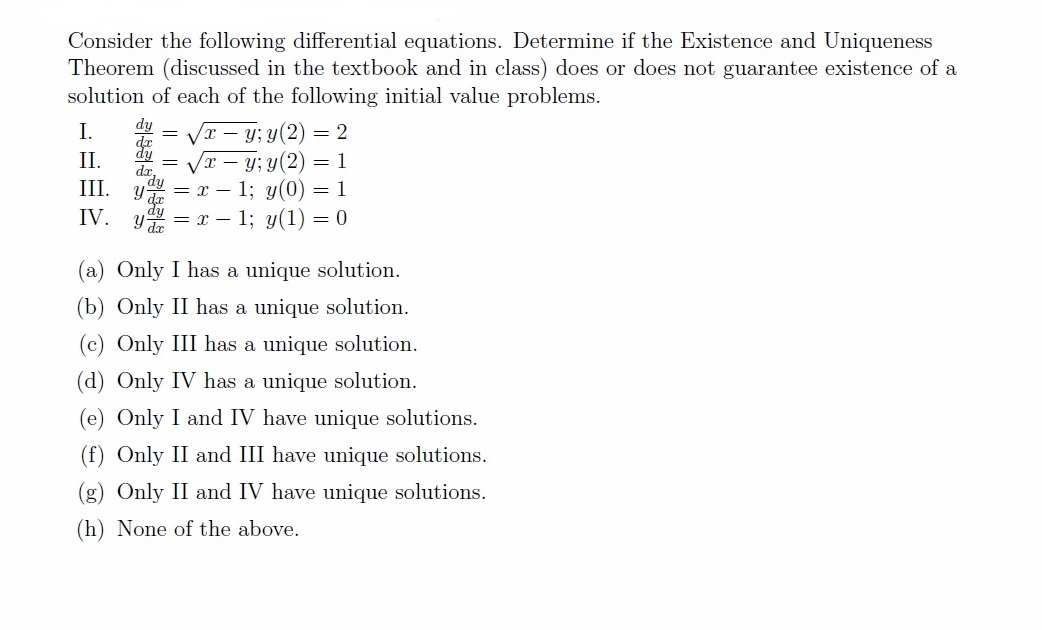
Solved Consider the following differential equations.
Then the differential equation (2) with initial con. Notes on the existence and uniqueness theorem for first order differential equations i. (a) is an existence theorem. It’s important to understand exactly what theorem 1.2.1 says. Let the function f(t,y) be continuous and satisfy the bound (3).
Solved (1 pt) Fundamental Existence Theorem for Linear
Notes on the existence and uniqueness theorem for first order differential equations i. It guarantees that a solution exists on. It’s important to understand exactly what theorem 1.2.1 says. Let the function f(t,y) be continuous and satisfy the bound (3). (a) is an existence theorem.
Differential Equations Existence and Uniqueness Theorem Is my answer
It’s important to understand exactly what theorem 1.2.1 says. Then the differential equation (2) with initial con. Let the function f(t,y) be continuous and satisfy the bound (3). It guarantees that a solution exists on. (a) is an existence theorem.
(A) Is An Existence Theorem.
Let the function f(t,y) be continuous and satisfy the bound (3). It guarantees that a solution exists on. Notes on the existence and uniqueness theorem for first order differential equations i. It’s important to understand exactly what theorem 1.2.1 says.