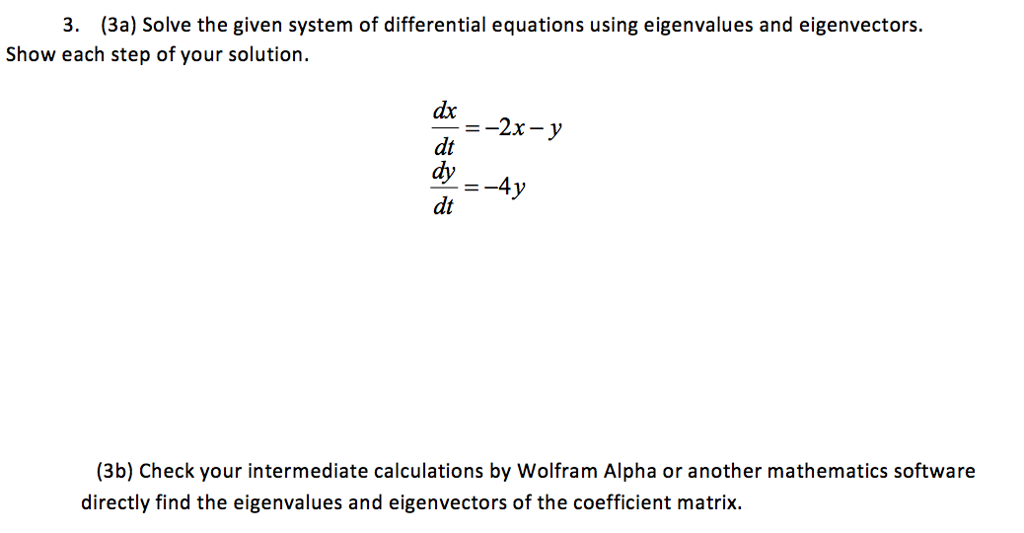
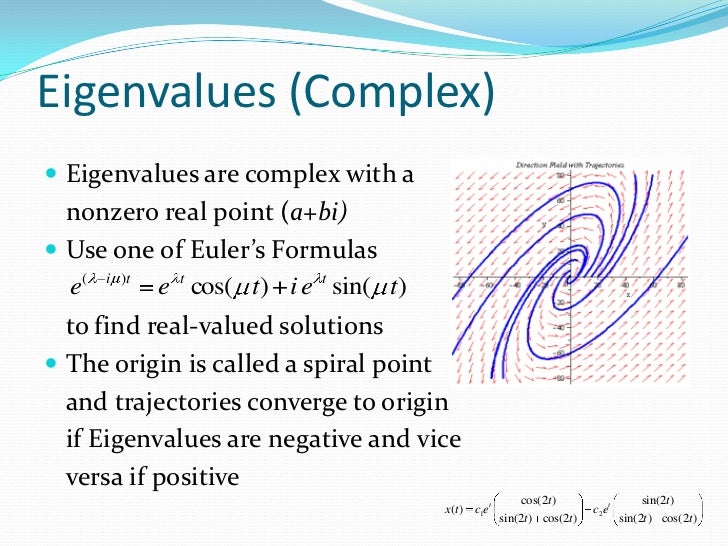
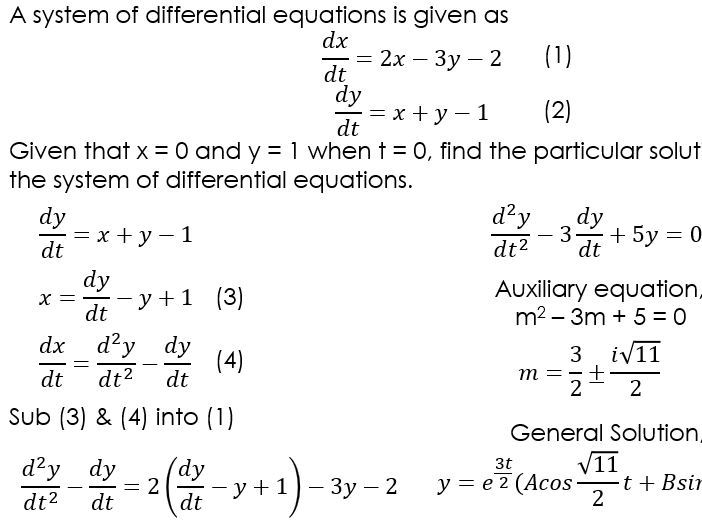Eigenvalues Differential Equations - Understanding eigenvalues and eigenvectors is essential for solving systems of differential equations, particularly in finding solutions to. Here is the eigenvalue and x is the eigenvector. This chapter ends by solving linear differential equations du/dt = au. The pieces of the solution are u(t) = eλtx instead of un =. In this section we will learn how to solve linear homogeneous constant coefficient systems of odes by the eigenvalue method. This is why we make the. Note that it is always true that a0 = 0 for any. In this section we will introduce the concept of eigenvalues and eigenvectors of a matrix. This section introduces eigenvalues and eigenvectors of a matrix, and discusses the role of the eigenvalues in determining the behavior of. We define the characteristic polynomial.
Here is the eigenvalue and x is the eigenvector. This is why we make the. This chapter ends by solving linear differential equations du/dt = au. Note that it is always true that a0 = 0 for any. This section introduces eigenvalues and eigenvectors of a matrix, and discusses the role of the eigenvalues in determining the behavior of. The pieces of the solution are u(t) = eλtx instead of un =. Understanding eigenvalues and eigenvectors is essential for solving systems of differential equations, particularly in finding solutions to. In this section we will introduce the concept of eigenvalues and eigenvectors of a matrix. We define the characteristic polynomial. In this section we will learn how to solve linear homogeneous constant coefficient systems of odes by the eigenvalue method.
Note that it is always true that a0 = 0 for any. In this section we will introduce the concept of eigenvalues and eigenvectors of a matrix. This section introduces eigenvalues and eigenvectors of a matrix, and discusses the role of the eigenvalues in determining the behavior of. We define the characteristic polynomial. In this section we will learn how to solve linear homogeneous constant coefficient systems of odes by the eigenvalue method. Understanding eigenvalues and eigenvectors is essential for solving systems of differential equations, particularly in finding solutions to. The pieces of the solution are u(t) = eλtx instead of un =. This is why we make the. This chapter ends by solving linear differential equations du/dt = au. Here is the eigenvalue and x is the eigenvector.
Solved Solve the given system of differential equations
This section introduces eigenvalues and eigenvectors of a matrix, and discusses the role of the eigenvalues in determining the behavior of. Understanding eigenvalues and eigenvectors is essential for solving systems of differential equations, particularly in finding solutions to. The pieces of the solution are u(t) = eλtx instead of un =. Here is the eigenvalue and x is the eigenvector..
Modelling with differential equations Teaching Resources
Understanding eigenvalues and eigenvectors is essential for solving systems of differential equations, particularly in finding solutions to. Here is the eigenvalue and x is the eigenvector. The pieces of the solution are u(t) = eλtx instead of un =. This is why we make the. Note that it is always true that a0 = 0 for any.
linear algebra Question about differential equations \tfrac {dx}{dt
This is why we make the. Note that it is always true that a0 = 0 for any. In this section we will introduce the concept of eigenvalues and eigenvectors of a matrix. Understanding eigenvalues and eigenvectors is essential for solving systems of differential equations, particularly in finding solutions to. The pieces of the solution are u(t) = eλtx instead.
linear algebra Using eigenvectors and values to get systems of
Understanding eigenvalues and eigenvectors is essential for solving systems of differential equations, particularly in finding solutions to. This is why we make the. In this section we will learn how to solve linear homogeneous constant coefficient systems of odes by the eigenvalue method. This section introduces eigenvalues and eigenvectors of a matrix, and discusses the role of the eigenvalues in.
Constant Coefficient Equations w Complex Roots constant coefficient
This section introduces eigenvalues and eigenvectors of a matrix, and discusses the role of the eigenvalues in determining the behavior of. Here is the eigenvalue and x is the eigenvector. In this section we will introduce the concept of eigenvalues and eigenvectors of a matrix. In this section we will learn how to solve linear homogeneous constant coefficient systems of.
Particular Solution of NonHomogeneous Differential Equations Mr
This chapter ends by solving linear differential equations du/dt = au. In this section we will learn how to solve linear homogeneous constant coefficient systems of odes by the eigenvalue method. Here is the eigenvalue and x is the eigenvector. We define the characteristic polynomial. Note that it is always true that a0 = 0 for any.
eigenvalues eigenvectors Differential Equations Direction Field
Note that it is always true that a0 = 0 for any. This is why we make the. This chapter ends by solving linear differential equations du/dt = au. Here is the eigenvalue and x is the eigenvector. In this section we will learn how to solve linear homogeneous constant coefficient systems of odes by the eigenvalue method.
Eigenvalues and Eigenvectors, Linear Differential Equations CSE 494
Understanding eigenvalues and eigenvectors is essential for solving systems of differential equations, particularly in finding solutions to. This section introduces eigenvalues and eigenvectors of a matrix, and discusses the role of the eigenvalues in determining the behavior of. Here is the eigenvalue and x is the eigenvector. Note that it is always true that a0 = 0 for any. This.
Systems of Differential Equations KZHU.ai 🚀
This section introduces eigenvalues and eigenvectors of a matrix, and discusses the role of the eigenvalues in determining the behavior of. This chapter ends by solving linear differential equations du/dt = au. In this section we will learn how to solve linear homogeneous constant coefficient systems of odes by the eigenvalue method. Understanding eigenvalues and eigenvectors is essential for solving.
Systems Of Differential Equations
This section introduces eigenvalues and eigenvectors of a matrix, and discusses the role of the eigenvalues in determining the behavior of. This is why we make the. This chapter ends by solving linear differential equations du/dt = au. The pieces of the solution are u(t) = eλtx instead of un =. In this section we will introduce the concept of.
Note That It Is Always True That A0 = 0 For Any.
We define the characteristic polynomial. This section introduces eigenvalues and eigenvectors of a matrix, and discusses the role of the eigenvalues in determining the behavior of. The pieces of the solution are u(t) = eλtx instead of un =. This chapter ends by solving linear differential equations du/dt = au.
In This Section We Will Learn How To Solve Linear Homogeneous Constant Coefficient Systems Of Odes By The Eigenvalue Method.
Understanding eigenvalues and eigenvectors is essential for solving systems of differential equations, particularly in finding solutions to. Here is the eigenvalue and x is the eigenvector. This is why we make the. In this section we will introduce the concept of eigenvalues and eigenvectors of a matrix.