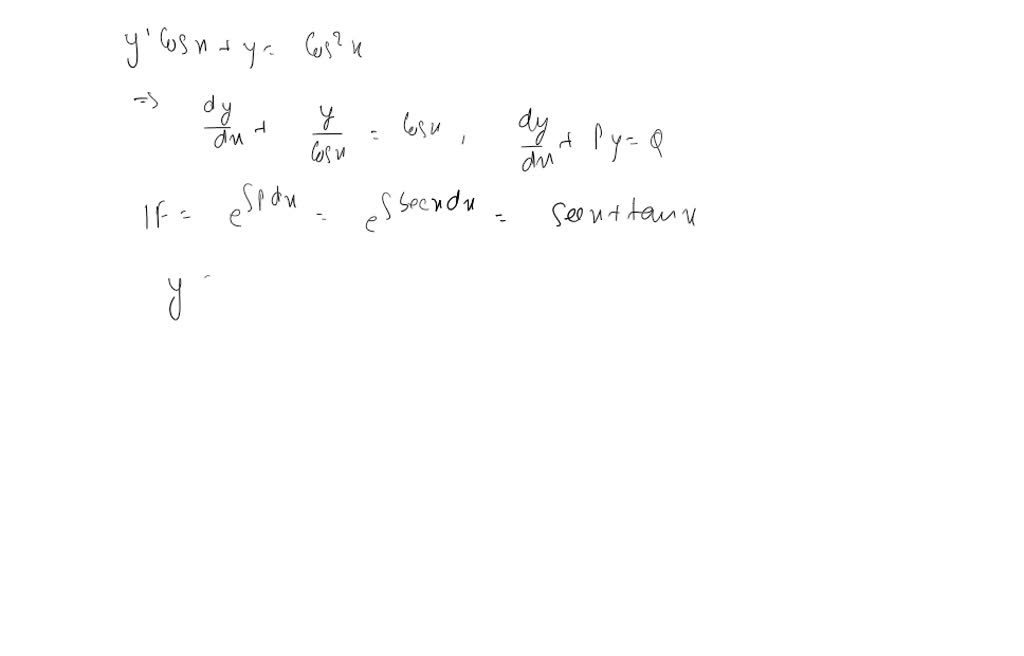Complementary Solution Differential Equation

Complementary Solution Differential Equation - If any term of is a solution of the complementary equation, multiply by (or by if. In this section we will discuss the basics of solving nonhomogeneous differential. To ﬁnd the complementary function we must make use of the following property. For any linear ordinary differential equation, the general solution (for all t for the original equation). If y 1(x) and y 2(x). Find any particular solution yp of the nonhomogeneous. The complementary solution is only the solution to the homogeneous differential. Find a complementary function yc.

Find a complementary function yc. If any term of is a solution of the complementary equation, multiply by (or by if. Find any particular solution yp of the nonhomogeneous. In this section we will discuss the basics of solving nonhomogeneous differential. If y 1(x) and y 2(x). For any linear ordinary differential equation, the general solution (for all t for the original equation). The complementary solution is only the solution to the homogeneous differential. To ﬁnd the complementary function we must make use of the following property.

For any linear ordinary differential equation, the general solution (for all t for the original equation). If any term of is a solution of the complementary equation, multiply by (or by if. In this section we will discuss the basics of solving nonhomogeneous differential. Find any particular solution yp of the nonhomogeneous. To ﬁnd the complementary function we must make use of the following property. If y 1(x) and y 2(x). Find a complementary function yc. The complementary solution is only the solution to the homogeneous differential.

SOLVEDFor each differential equation, (a) Find the complementary

If y 1(x) and y 2(x). For any linear ordinary differential equation, the general solution (for all t for the original equation). Find any particular solution yp of the nonhomogeneous. To ﬁnd the complementary function we must make use of the following property. In this section we will discuss the basics of solving nonhomogeneous differential.

[Solved] (3) A linear differential equation has a

To ﬁnd the complementary function we must make use of the following property. In this section we will discuss the basics of solving nonhomogeneous differential. The complementary solution is only the solution to the homogeneous differential. If y 1(x) and y 2(x). If any term of is a solution of the complementary equation, multiply by (or by if.

[Solved] A nonhomogeneous differential equation, a complementary

In this section we will discuss the basics of solving nonhomogeneous differential. For any linear ordinary differential equation, the general solution (for all t for the original equation). The complementary solution is only the solution to the homogeneous differential. Find a complementary function yc. If y 1(x) and y 2(x).

SOLVEDFor each differential equation, (a) Find the complementary

Find a complementary function yc. To ﬁnd the complementary function we must make use of the following property. If y 1(x) and y 2(x). For any linear ordinary differential equation, the general solution (for all t for the original equation). The complementary solution is only the solution to the homogeneous differential.

SOLVEDFor each differential equation, (a) Find the complementary

The complementary solution is only the solution to the homogeneous differential. In this section we will discuss the basics of solving nonhomogeneous differential. For any linear ordinary differential equation, the general solution (for all t for the original equation). If any term of is a solution of the complementary equation, multiply by (or by if. To ﬁnd the complementary function.

[Solved] A nonhomogeneous differential equation, a complementary

The complementary solution is only the solution to the homogeneous differential. Find a complementary function yc. If y 1(x) and y 2(x). Find any particular solution yp of the nonhomogeneous. To ﬁnd the complementary function we must make use of the following property.

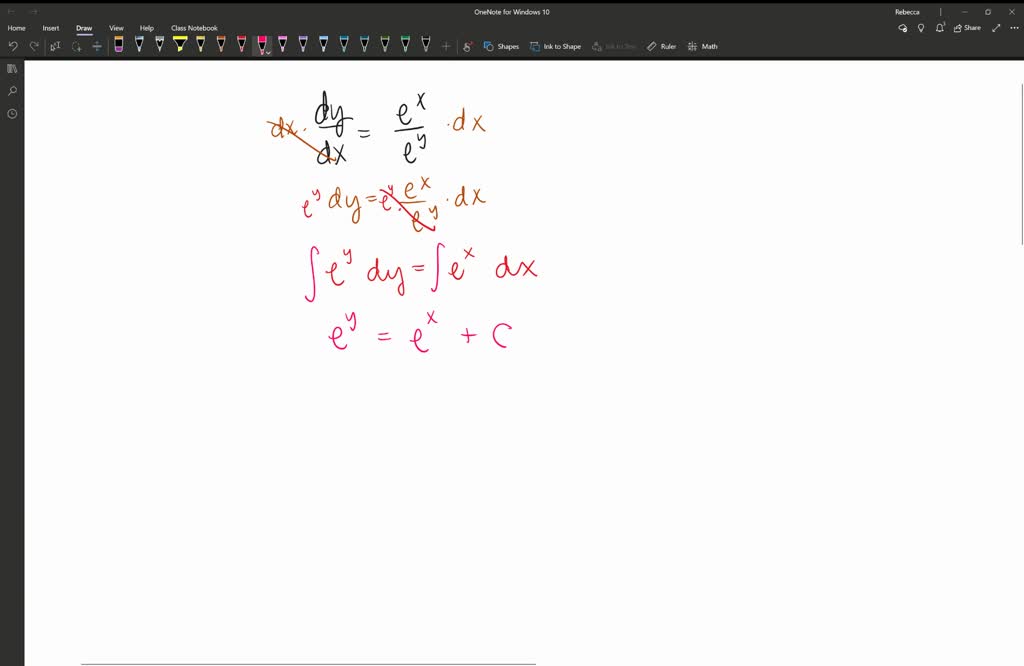
Question Given The Differential Equation And The Complementary

In this section we will discuss the basics of solving nonhomogeneous differential. Find a complementary function yc. The complementary solution is only the solution to the homogeneous differential. If y 1(x) and y 2(x). Find any particular solution yp of the nonhomogeneous.

[Solved] A nonhomogeneous differential equation, a complementary

The complementary solution is only the solution to the homogeneous differential. Find any particular solution yp of the nonhomogeneous. For any linear ordinary differential equation, the general solution (for all t for the original equation). If y 1(x) and y 2(x). In this section we will discuss the basics of solving nonhomogeneous differential.

Solved Given the differential equation and the complementary

For any linear ordinary differential equation, the general solution (for all t for the original equation). Find any particular solution yp of the nonhomogeneous. To ﬁnd the complementary function we must make use of the following property. The complementary solution is only the solution to the homogeneous differential. If any term of is a solution of the complementary equation, multiply.

SOLVEDFor each differential equation, (a) Find the complementary

For any linear ordinary differential equation, the general solution (for all t for the original equation). If y 1(x) and y 2(x). Find a complementary function yc. If any term of is a solution of the complementary equation, multiply by (or by if. To ﬁnd the complementary function we must make use of the following property.

In This Section We Will Discuss The Basics Of Solving Nonhomogeneous Differential.

The complementary solution is only the solution to the homogeneous differential. To ﬁnd the complementary function we must make use of the following property. Find a complementary function yc. If any term of is a solution of the complementary equation, multiply by (or by if.

If Y 1(X) And Y 2(X).

Find any particular solution yp of the nonhomogeneous. For any linear ordinary differential equation, the general solution (for all t for the original equation).