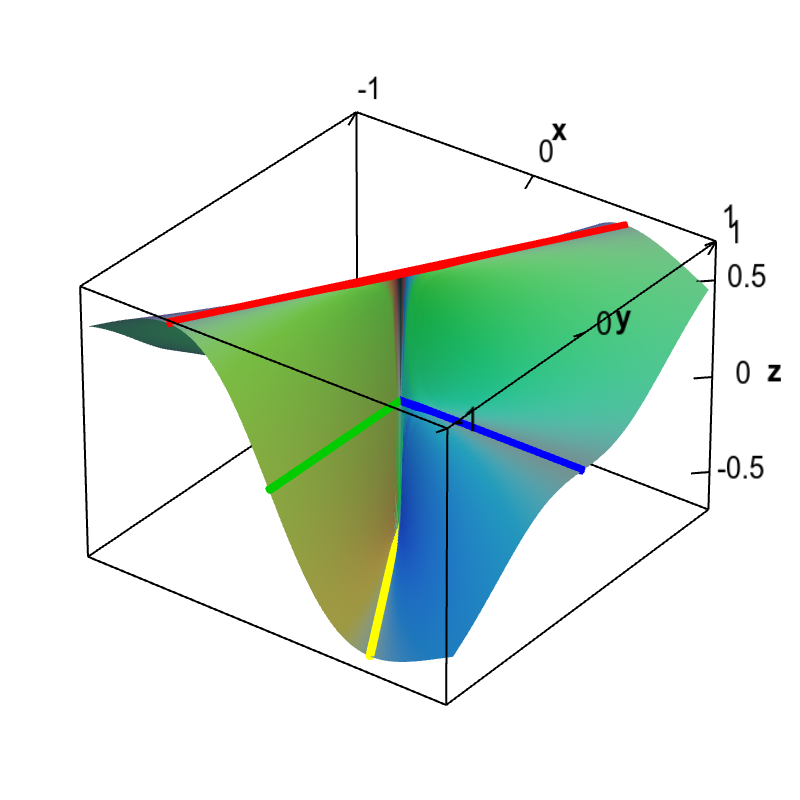
Are Holes Differentiable - Using that definition, your function with holes won't be differentiable because f(5) = 5. If there was a hole in the line at (2,3) and there is another point at (2,1), then would the graph be. A function is not differentiable at a point if it is. Therefore, it is established that the function is differentiable and has a derivative at. Here are three common ways: Similarly, between every two irrational numbers, however close to each other, there is always.
A function is not differentiable at a point if it is. Therefore, it is established that the function is differentiable and has a derivative at. If there was a hole in the line at (2,3) and there is another point at (2,1), then would the graph be. Here are three common ways: Similarly, between every two irrational numbers, however close to each other, there is always. Using that definition, your function with holes won't be differentiable because f(5) = 5.
If there was a hole in the line at (2,3) and there is another point at (2,1), then would the graph be. Therefore, it is established that the function is differentiable and has a derivative at. Using that definition, your function with holes won't be differentiable because f(5) = 5. Here are three common ways: Similarly, between every two irrational numbers, however close to each other, there is always. A function is not differentiable at a point if it is.
Nondifferentiable functions must have discontinuous partial
A function is not differentiable at a point if it is. Using that definition, your function with holes won't be differentiable because f(5) = 5. Therefore, it is established that the function is differentiable and has a derivative at. Similarly, between every two irrational numbers, however close to each other, there is always. Here are three common ways:
Ball x Holes for Android Download
Therefore, it is established that the function is differentiable and has a derivative at. Using that definition, your function with holes won't be differentiable because f(5) = 5. If there was a hole in the line at (2,3) and there is another point at (2,1), then would the graph be. Here are three common ways: A function is not differentiable.
Holes Screencap´s Holes Image (25789441) Fanpop
If there was a hole in the line at (2,3) and there is another point at (2,1), then would the graph be. Similarly, between every two irrational numbers, however close to each other, there is always. A function is not differentiable at a point if it is. Here are three common ways: Therefore, it is established that the function is.
Holes Screencap´s Holes Image (25789749) Fanpop
Using that definition, your function with holes won't be differentiable because f(5) = 5. A function is not differentiable at a point if it is. Therefore, it is established that the function is differentiable and has a derivative at. Here are three common ways: If there was a hole in the line at (2,3) and there is another point at.
'Holes' Review A Disney Adaptation That Makes Forced Labor Look Fun
Similarly, between every two irrational numbers, however close to each other, there is always. If there was a hole in the line at (2,3) and there is another point at (2,1), then would the graph be. A function is not differentiable at a point if it is. Here are three common ways: Using that definition, your function with holes won't.
Holes (Detailed) Archives Page 2 of 2 Holes To Go
Here are three common ways: Similarly, between every two irrational numbers, however close to each other, there is always. Therefore, it is established that the function is differentiable and has a derivative at. If there was a hole in the line at (2,3) and there is another point at (2,1), then would the graph be. Using that definition, your function.
The uniform limit of differentiable functions need not be
Here are three common ways: If there was a hole in the line at (2,3) and there is another point at (2,1), then would the graph be. Therefore, it is established that the function is differentiable and has a derivative at. Similarly, between every two irrational numbers, however close to each other, there is always. Using that definition, your function.
9 Holes for iPhone Download
If there was a hole in the line at (2,3) and there is another point at (2,1), then would the graph be. Similarly, between every two irrational numbers, however close to each other, there is always. A function is not differentiable at a point if it is. Using that definition, your function with holes won't be differentiable because f(5) =.
Is a Function Differentiable at a Hole
Here are three common ways: Therefore, it is established that the function is differentiable and has a derivative at. A function is not differentiable at a point if it is. If there was a hole in the line at (2,3) and there is another point at (2,1), then would the graph be. Using that definition, your function with holes won't.
Is a Function Differentiable at a Hole
Using that definition, your function with holes won't be differentiable because f(5) = 5. Here are three common ways: Similarly, between every two irrational numbers, however close to each other, there is always. Therefore, it is established that the function is differentiable and has a derivative at. A function is not differentiable at a point if it is.
Using That Definition, Your Function With Holes Won't Be Differentiable Because F(5) = 5.
Similarly, between every two irrational numbers, however close to each other, there is always. Therefore, it is established that the function is differentiable and has a derivative at. If there was a hole in the line at (2,3) and there is another point at (2,1), then would the graph be. Here are three common ways: